Let f(x)=x^(3)+9x^(2)+13 x and let c be the number that satisfies the Mean Value Theorem for f on the interval -7

Question

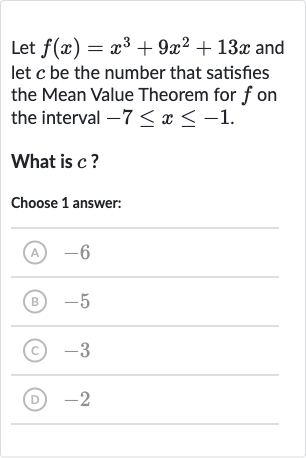
Let  f(x)=x^(3)+9x^(2)+13 x and let  c be the number that satisfies the Mean Value Theorem for  f on the interval  -7 <= x <= -1. What is  c ? Choose 1 answer: (A) -6 (B) -5 (C) -3 (D) -2

Bytelearn · Accepted Answer

Mean Value Theorem Explanation: The Mean Value Theorem states that if a function f is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one number c in the interval (a, b) such that f'(c) is equal to the average rate of change of the function over [a, b]. This can be written as:  f'(c) = (f(b) - f(a)) / (b - a)  Given the function f(x) = x^3 + 9x^2 + 13x, we need to find the derivative f'(x) to apply the Mean Value Theorem.Finding Derivative: To find f'(x), we differentiate f(x) with respect to x:  f'(x) = d/dx (x^3 + 9x^2 + 13x)        = 3x^2 + 18x + 13  Now we have the derivative of the function.Calculating Average Rate of Change: Next, we need to calculate the average rate of change of f(x) over the interval [-7, -1]. This requires evaluating f(x) at the endpoints of the interval:  f(-7) = (-7)^3 + 9(-7)^2 + 13(-7)       = -343 + 441 - 91       = -343 + 350       = 7  f(-1) = (-1)^3 + 9(-1)^2 + 13(-1)        = -1 + 9 - 13        = -5  Now we have the values of f(x) at the endpoints of the interval.Applying Mean Value Theorem: We can now calculate the average rate of change of f(x) over the interval [-7, -1]:  (f(-1) - f(-7)) / (-1 - (-7)) = (-5 - 7) / (-1 + 7) = -12 / 6 = -2  The average rate of change of f(x) over the interval is -2.Solving Quadratic Equation: According to the Mean Value Theorem, there exists a number c in the interval (-7, -1) such that f'(c) = -2. We have already found that f'(x) = 3x^2 + 18x + 13. We set this equal to -2 to find c:  3c^2 + 18c + 13 = -2  Now we need to solve this quadratic equation for c.Using Quadratic Formula: We rearrange the equation to bring all terms to one side:  3c^2 + 18c + 13 + 2 = 0 3c^2 + 18c + 15 = 0  Now we have a standard quadratic equation to solve for c.Finding Valid Solution: To solve the quadratic equation, we can either factor it, complete the square, or use the quadratic formula. The quadratic formula is:  c = (-b ± √(b^2 - 4ac)) / (2a)  For our equation, a = 3, b = 18, and c = 15. We substitute these values into the formula to find the roots.Using the quadratic formula:  c = (-18 ± √(18^2 - 4*3*15)) / (2*3) c = (-18 ± √(324 - 180)) / 6 c = (-18 ± √144) / 6 c = (-18 ± 12) / 6  This gives us two possible solutions for c.The two possible solutions for c are:  c = (-18 + 12) / 6 = -6 / 6 = -1 c = (-18 - 12) / 6 = -30 / 6 = -5  However, c must be in the interval (-7, -1), so c = -1 is not a valid solution because it is not in the open interval. Therefore, the only valid solution is c = -5.

Let $f(x)=x^{3}+9 x^{2}+13 x$ and let $c$ be the number that satisfies the Mean Value Theorem for $f$ on the interval $-7 \leq x \leq-1$ . $\newline$ What is $c$ ? $\newline$ Choose $1$ answer: $\newline$ (A) $-6$ $\newline$ (B) $-5$ $\newline$ (C) $-3$ $\newline$ (D) $-2$

Full solution

More problems from Euler's method

Related topics