AI tutor
Full solution
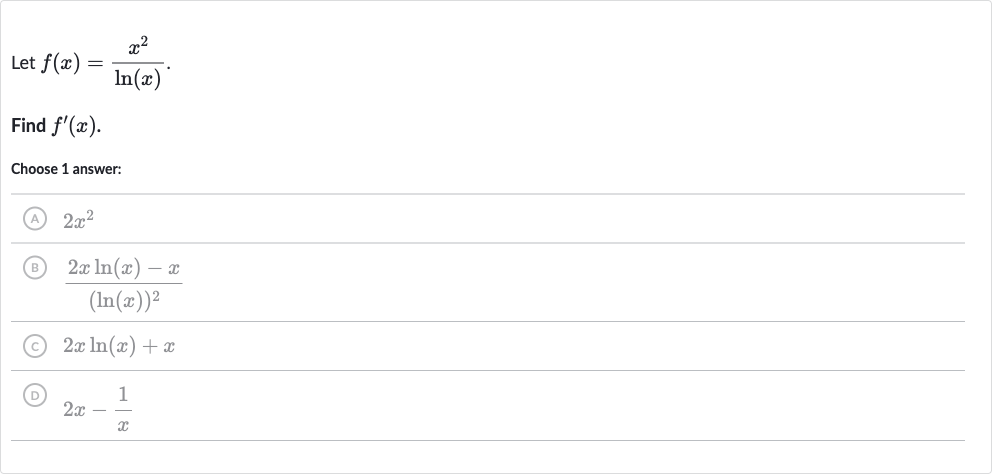
Q. Let .Find .Choose answer:(A) (B) (C) (D)
- Use Quotient Rule: To find the derivative of the function , we will use the quotient rule, which states that if we have a function , then . Here, and .
- Find : First, we find the derivative of . The derivative of with respect to is .
- Find : Next, we find the derivative of . The derivative of with respect to is .
- Apply Quotient Rule: Now we apply the quotient rule. We have and . Plugging these into the quotient rule formula, we get:
- Simplify Numerator: Simplify the expression inside the numerator of the derivative: .
- Match Answer Choice: We can see that the expression matches answer choice (B), which is .