Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

What is the period of

y=8cos(5pi x+(3pi)/(2))-9?
Give an exact value.
units

What is the period of $\newline$ $y=8 \cos \left(5 \pi x+\frac{3 \pi}{2}\right)-9 ?$ $\newline$ Give an exact value. $\newline$ units

View step-by-step help

View step-by-step help

Find the sum of a finite geometric series

Full solution

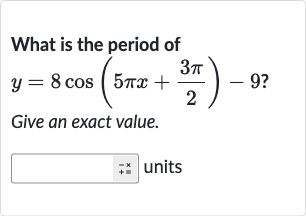
Q. What is the period of

\newline

y=8 \cos \left(5 \pi x+\frac{3 \pi}{2}\right)-9 ?

\newline

Give an exact value.

\newline

units

Formula for cosine function period: The period of a cosine function of the form $y = A \cos(Bx + C) + D$ is given by the formula Period = $\frac{2\pi}{|B|}$ . Here, $A$ is the amplitude, $B$ is the frequency, $C$ is the phase shift, and $D$ is the vertical shift.
Identifying the value of $B$ : Identify the value of $B$ in the given function $y =$ $8$ \cos( $5$ \pi x + \frac{ $3$ \pi}{ $2$ }) - $9$ . The value of $B$ is the coefficient of $x$ inside the cosine function, which is $5$ \pi.
Calculating the period: Calculate the period using the formula $\text{Period} = \frac{2\pi}{|B|}$ . Substitute $B$ with $5\pi$ . $\newline$ $\text{Period} = \frac{2\pi}{|5\pi|} = \frac{2\pi}{5\pi}$ .
Simplifying the expression: Simplify the expression for the period. Since $\pi$ is in both the numerator and the denominator, they cancel each other out. $\newline$ Period = $\frac{2}{5}$ .
Exact value of the period: The exact value of the period of the function $y = 8\cos(5\pi x + \frac{3\pi}{2}) - 9$ is $\frac{2}{5}$ units.

More problems from Find the sum of a finite geometric series

Question

What is the period of

\newline

y=-2 \sin \left(\frac{2}{3} x-4\right) ?

\newline

Give an exact value.

\newline

Posted 3 months ago

Question

z=91-27 i

\newline

What is the real part of

z

\newline

What is the imaginary part of

z

Posted 3 months ago

Question

z=-19 i+14

\newline

What is the real part of

z

\newline

What is the imaginary part of

z

Posted 3 months ago

Question

z=-302+19.3 i

\newline

What is the real part of

z

\newline

What is the imaginary part of

z

Posted 3 months ago

Question

The sum of the first

3

terms of a geometric series is

171

and the common ratio is

\frac{2}{3}

\newline

What is the first term of the series?

Posted 3 months ago

Question

The first term in a geometric series is

5

and the common ratio is

2

\newline

Find the sum of the first

10

terms in the series.

Posted 3 months ago

Question

What happens to the value of the expression

20+a

a

\newline

1

\newline

(A) It increases.

\newline

(B) It decreases.

\newline

(C) It stays the same.

Posted 3 months ago

Question

What is the constant of proportionality in the equation

y=7 x

\newline

constant of proportionality

=

Posted 3 months ago

Question

What is the constant of proportionality in the equation

y=3 x

\newline

constant of proportionality

=

Posted 3 months ago

Question

Isabel tried to evaluate an expression step by step.

(2+8)+8

. Find Isabel's first mistake

Posted 13 hours ago