The sum of the first $3$ terms of a geometric series is $171$ and the common ratio is $\frac{2}{3}$ . $\newline$ What is the first term of the series?

View step-by-step help

Home

Math Problems

Algebra 2

Find the sum of a finite geometric series

Full solution

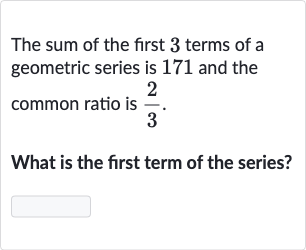
Q. The sum of the first

3

terms of a geometric series is

171

and the common ratio is

\frac{2}{3}

\newline

What is the first term of the series?

Geometric series sum formula: The sum of the first $n$ terms of a geometric series is given by the formula: $\newline$ $S_n = \frac{a_1(1 - r^n)}{1 - r}$ $\newline$ where $S_n$ is the sum of the first $n$ terms, $a_1$ is the first term, $r$ is the common ratio, and $n$ is the number of terms. $\newline$ In this problem, we have: $\newline$ $S_3 = 171$ $\newline$ $r = \frac{2}{3}$ $\newline$ $n = 3$ $\newline$ We need to find $a_1$ .
Substituting known values: Let's substitute the known values into the sum formula and solve for $a_1$ . $\newline$ $171 = a_1\left(1 - \left(\frac{2}{3}\right)^3\right) / \left(1 - \frac{2}{3}\right)$ $\newline$ Now we need to calculate $\left(\frac{2}{3}\right)^3$ and simplify the equation.
Calculating $(\frac{2}{3})^3$ : Calculate $(\frac{2}{3})^3$ : $\newline$ $(\frac{2}{3})^3 = \frac{2^3}{3^3} = \frac{8}{27}$ $\newline$ Now substitute this value into the equation: $\newline$ $171 = a_1(1 - \frac{8}{27}) / (1 - \frac{2}{3})$
Simplifying the equation: Simplify the equation further: $\newline$ $171 = a_1\left(1 - \frac{8}{27}\right) / \left(\frac{1}{3}\right)$ $\newline$ $171 = a_1\left(\frac{27}{27} - \frac{8}{27}\right) / \left(\frac{1}{3}\right)$ $\newline$ $171 = a_1\left(\frac{19}{27}\right) \cdot 3$
Isolating $a_1$ : Multiply both sides of the equation by $\frac{27}{19}$ to isolate $a_1$ : $\newline$ $171 \times \left(\frac{27}{19}\right) = a_1 \times 3$ $\newline$ Now we need to calculate $171 \times \left(\frac{27}{19}\right)$ .
Calculating $171 \times \left(\frac{27}{19}\right)$ : Calculate $171 \times \left(\frac{27}{19}\right)$ : $\newline$ $171 \times \left(\frac{27}{19}\right) = 9 \times 19 \times \left(\frac{27}{19}\right) = 9 \times 27$ $\newline$ Now we have: $\newline$ $9 \times 27 = a_1 \times 3$
Solving for $a_1$ : Divide both sides by $3$ to solve for $a_1$ : $\newline$ $9 \times 27 / 3 = a_1$ $\newline$ Calculate $9 \times 27 / 3$ : $\newline$ $9 \times 27 / 3 = 9 \times 9 = 81$ $\newline$ So, $a_1 = 81$