AI tutor
Full solution
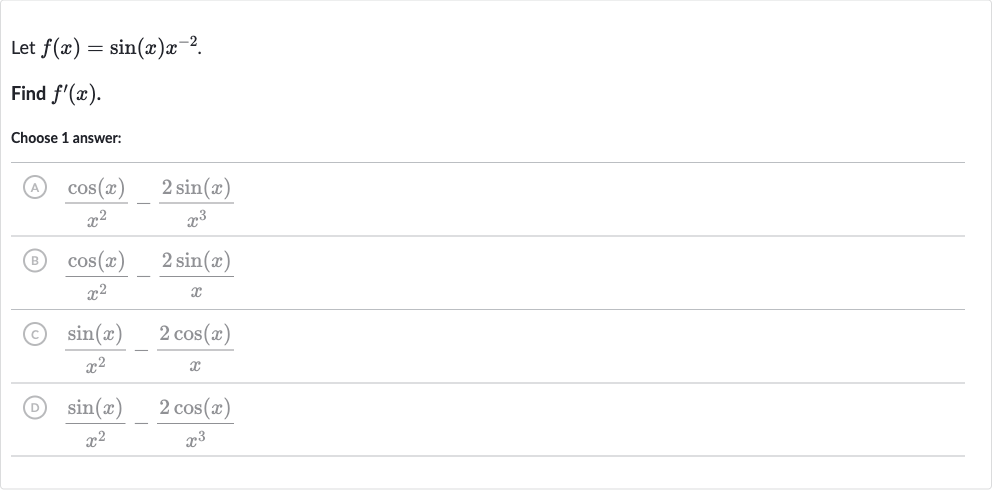
Q. Let .Find .Choose answer:(A) (B) (C) (D)
- Apply Product Rule: Use the product rule for differentiation: , where and .
- Differentiate : Differentiate . The derivative of with respect to is , so .
- Differentiate : Differentiate . The derivative of with respect to is , so .
- Apply Product Rule: Apply the product rule: .
- Simplify Expression: Simplify the expression: .