If $y$ varies inversely as the $4$ th power of $x$ and $y=2$ when $x=-1$ , what is $y$ when $x=0.5$ ?

View step-by-step help

Home

Math Problems

Algebra 1

Write and solve inverse variation equations

Full solution

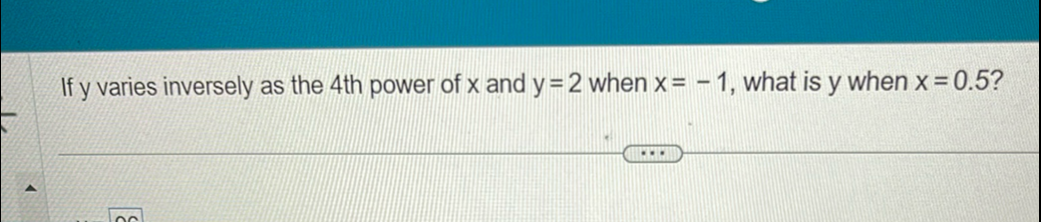
Q. If

y

varies inversely as the

4

th power of

x

and

y=2

when

x=-1

, what is

y

when

x=0.5

Identify general form of inverse variation: Given that $y$ varies inversely as the $4$ th power of $x$ . Identify the general form of inverse variation when it involves a power. Inverse variation with a power: $y = \frac{k}{x^n}$ where $n$ is the power. Here, $n = 4$ , so the equation becomes $y = \frac{k}{x^4}$ .
Substitute values in equation: We know that $y = 2$ when $x = -1$ . Choose the equation after substituting the values in $y = \frac{k}{x^4}$ . Substitute $-1$ for $x$ and $2$ for $y$ in $y = \frac{k}{x^4}$ . $2 = \frac{k}{(-1)^4}$ Since $(-1)^4 = 1$ , the equation simplifies to $x = -1$ $0$ .
Solve for $k$ : We found: $\newline$ $2 = \frac{k}{1}$ $\newline$ Solve the equation to find the value of $k$ . $\newline$ To isolate $k$ , multiply both sides by $1$ . $\newline$ $2 \times 1 = k$ $\newline$ $k = 2$
Write inverse variation equation: We have: $\newline$ $k = 2$ $\newline$ Write the inverse variation equation in the form of $y = \frac{k}{x^4}$ . $\newline$ Substitute $k = 2$ in $y = \frac{k}{x^4}$ . $\newline$ $y = \frac{2}{x^4}$
Find $y$ for $x=0.5$ : Inverse variation equation: $\newline$ $y = \frac{2}{x^4}$ $\newline$ Find $y$ when $x = 0.5$ . $\newline$ Substitute $0.5$ for $x$ in $y = \frac{2}{x^4}$ . $\newline$ $y = \frac{2}{(0.5)^4}$ $\newline$ Calculate $(0.5)^4$ . $\newline$ $x=0.5$ $0$
Find $y$ for $x=0.5$ : Inverse variation equation: $\newline$ $y = \frac{2}{x^4}$ $\newline$ Find $y$ when $x = 0.5$ . $\newline$ Substitute $0.5$ for $x$ in $y = \frac{2}{x^4}$ . $\newline$ $y = \frac{2}{(0.5)^4}$ $\newline$ Calculate $(0.5)^4$ . $\newline$ $x=0.5$ $0$ Substitute $x=0.5$ $1$ for $(0.5)^4$ in the equation. $\newline$ $x=0.5$ $3$ $\newline$ Calculate $x=0.5$ $4$ . $\newline$ $x=0.5$ $5$