AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
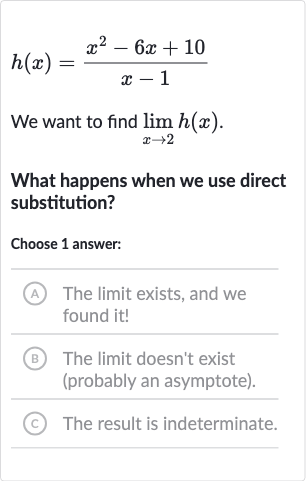
We want to find .What happens when we use direct substitution?Choose answer:(A) The limit exists, and we found it!(B) The limit doesn't exist (probably an asymptote).(C) The result is indeterminate.
Full solution
Q. We want to find .What happens when we use direct substitution?Choose answer:(A) The limit exists, and we found it!(B) The limit doesn't exist (probably an asymptote).(C) The result is indeterminate.
- Step : Direct Substitution: First, let's try direct substitution by plugging into the function .
- Step : Calculation: Now, let's perform the calculations.
- Step : Simplification: Since we were able to find a value by direct substitution without encountering division by zero or an indeterminate form, the limit exists.The limit as approaches for the function is .
More problems from Write and solve inverse variation equations
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
In the given equation, is a constant. If the equation has the solutions and , what is the value of ?
Get tutor help