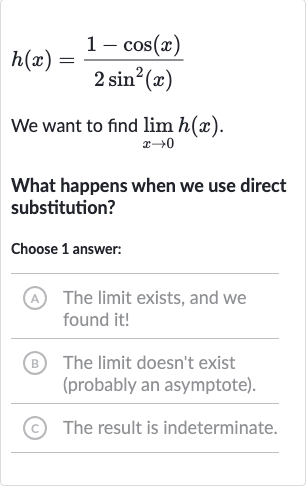
h(x)=(1-cos(x))/(2sin^(2)(x)) We want to find lim_(x rarr0)h(x). What happens when we use direct substitution? Choose 1 answer: (A) The limit exists, and we found it! (B) The limit doesn't exist (probably an asymptote). (C) The result is indeterminate.

Question

h(x)=(1-cos(x))/(2sin^(2)(x)) We want to find  lim_(x rarr0)h(x). What happens when we use direct substitution? Choose 1 answer: (A) The limit exists, and we found it! (B) The limit doesn't exist (probably an asymptote). (C) The result is indeterminate.

Bytelearn · Accepted Answer

Direct Substitution: Given the function h(x) = (1 - cos(x)) / (2sin^2(x)), we want to find the limit as x approaches 0. Let's start by using direct substitution to see what happens when we plug in x = 0 into the function. Direct substitution: h(0) = (1 - cos(0)) / (2sin^2(0)) Calculate the values of cosine and sine at x = 0. cos(0) = 1 and sin(0) = 0 Substitute these values into the function. h(0) = (1 - 1) / (2 * 0^2) h(0) = 0 / 0Indeterminate Form: The result of the direct substitution is 0/0, which is an indeterminate form. This means that we cannot determine the limit by direct substitution, and we need to apply other techniques to evaluate the limit.Using Trigonometric Identities: Since we have an indeterminate form, we need to manipulate the expression to find a way to evaluate the limit. One common technique is to use trigonometric identities to simplify the expression. We can use the identity sin^2(x) = (1 - cos(2x))/2 to rewrite the denominator. Rewrite the function using the identity. h(x) = (1 - cos(x)) / (2 * (1 - cos(2x))/2) Simplify the expression. h(x) = (1 - cos(x)) / (1 - cos(2x))Correcting the Identity: We made a mistake in the previous step. The correct identity for sin^2(x) is not (1 - cos(2x))/2, but rather (1 - cos^2(x))/2. We need to correct this and use the correct identity to simplify the expression.

Full solution

More problems from Write and solve inverse variation equations

Related topics