Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Given that
f(x)=x+4,quad g(x)=4x and
h(x)=2f(x-1)+3g(x), then what is the value of
h(1) ?
Answer:

Given that $f(x)=x+4, \quad g(x)=4 x$ and $h(x)=2 f(x-1)+3 g(x)$ , then what is the value of $h(1)$ ? $\newline$ Answer:

View step-by-step help

View step-by-step help

Write and solve inverse variation equations

Full solution

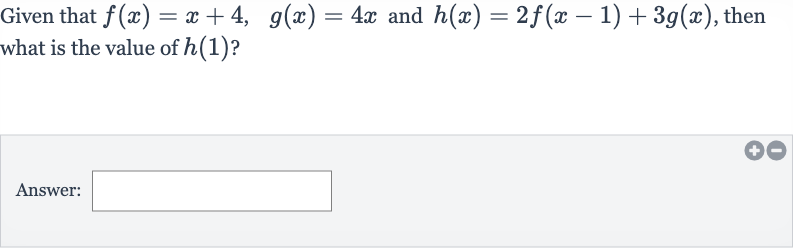
Q. Given that

f(x)=x+4, \quad g(x)=4 x

and

h(x)=2 f(x-1)+3 g(x)

, then what is the value of

h(1)

?

\newline

Answer:

Given Functions: Given the functions $f(x) = x + 4$ , $g(x) = 4x$ , and $h(x) = 2f(x - 1) + 3g(x)$ , we need to find the value of $h(1)$ . Let's start by finding the value of $f(x - 1)$ and $g(x)$ when $x = 1$ .
Calculate $f(0)$ : First, we calculate $f(1 - 1)$ which is $f(0)$ . Using the definition of $f(x)$ , we have $f(0) = 0 + 4 = 4$ .
Calculate $g(1)$ : Next, we calculate $g(1)$ using the definition of $g(x)$ . We have $g(1) = 4 \times 1 = 4$ .
Substitute into $h(1)$ : Now we have both $f(0)$ and $g(1)$ , we can substitute these values into the definition of $h(x)$ to find $h(1)$ . So, $h(1) = 2f(1 - 1) + 3g(1) = 2f(0) + 3g(1)$ .
Final Calculation: Substitute the values we found into the equation for $h(1)$ : $h(1) = 2 \times 4 + 3 \times 4 = 8 + 12 = 20$ .

More problems from Write and solve inverse variation equations

Question

In a direct variation,

y = 6

x = 2

. Write a direct variation equation that shows the relationship between

x

y

\newline

Write your answer as an equation with

y

first, followed by an equals sign.

Posted 5 months ago

Question

y

varies directly with

x

y = 12

x = 6

y

x = 4

\newline

Write and solve a direct variation equation to find the answer.

\newline

y =

Posted 5 months ago

Question

Which equation shows inverse variation?

\newline

\newline

(\frac{x}{36}) = y

\newline

yx = -74

Posted 5 months ago

Question

y

varies inversely with

x

y = 1

x = 4

y

x = 2

\newline

Write and solve an inverse variation equation to find the answer.

\newline

y =

Posted 5 months ago

Question

What is the value of

\frac{x^{2}}{y^{4}}

x=8

y=2

Posted 5 months ago

Question

(x+4)(2 x-a)=0

\newline

In the given equation,

a

is a constant. If the equation has the solutions

x=4

x=-4

, what is the value of

a

Posted 3 months ago

Question

(2 x+5)(-m x+9)=0

\newline

In the given equation,

m

is a constant. If the equation has the solutions

x=-\frac{5}{2}

x=\frac{3}{2}

, what is the value of

m

Posted 3 months ago

Question

(4 x+3)-(5 x-7)=a x+b

\newline

In the given equation,

b

is a constant. What is the value of

b

Posted 3 months ago

Question

y=\frac{29}{8} x-\frac{2}{3}

\newline

The given equation is graphed in the

x y

-plane. What is the slope of the line?

Posted 8 months ago

Question

a t^{2}+\frac{7}{2} t-4=0

\newline

The given equation has solutions at

t=-8

t=1

. What is the value of the constant

a

Posted 8 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant