Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the derivative of the following function.

y=e^(-5x^(5))
Answer:
y^(')=

Find the derivative of the following function. $\newline$ $y=e^{-5 x^{5}}$ $\newline$ Answer: $y^{\prime}=$

View step-by-step help

View step-by-step help

Find derivatives using logarithmic differentiation

Full solution

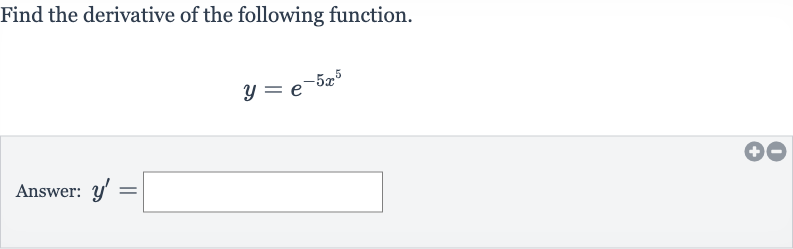
Q. Find the derivative of the following function.

\newline

y=e^{-5 x^{5}}

\newline

Answer:

y^{\prime}=

Identify Function and Rule: Identify the function and the rule to use for differentiation. $\newline$ We have the function $y = e^{-5x^{5}}$ . To find the derivative $y'$ , we will use the chain rule, which states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function.
Apply Chain Rule: Apply the chain rule to differentiate the function. $\newline$ The outer function is $e^u$ where $u = -5x^{5}$ . The derivative of $e^u$ with respect to $u$ is $e^u$ . The inner function is $u = -5x^{5}$ , and its derivative with respect to $x$ is $-5 \times 5x^{5-1} = -25x^{4}$ .
Multiply Derivatives: Multiply the derivatives of the outer and inner functions. $\newline$ $y' = e^{-5x^{5}} \times (-25x^{4})$
Simplify Expression: Simplify the expression if necessary. $\newline$ The expression is already simplified, so we have the final answer. $\newline$ $y' = -25x^{4}e^{-5x^{5}}$

More problems from Find derivatives using logarithmic differentiation

Question

\newline

\sqrt{\frac{9}{25}}

\newline

Posted 2 months ago

Question

\newline

1,000

\text{ milligrams}

\_\_\_\_

\text{ grams}

Posted 2 months ago

Question

3

-gallon bottle of bleach costs

\$11.52

. What is the price per quart?

\newline

Posted 2 months ago

Question

Maureen can't wait to make chocolate chip zucchini bread with zucchini from her garden. Her recipe calls for

8

ounces of chocolate chips per loaf. If she has enough zucchini to make

4

loaves of zucchini bread, how many pounds of chocolate chips does she need?

\newline

Hint: There are

16

1

\newline

Write your answer as a whole number, decimal, or simplified fraction. Do not round.

\newline

Posted 2 months ago

Question

A whale is swimming due north at a speed of

30

miles per hour. Just

5

miles away, a whale-watching tour boat is traveling south, directly toward the whale, at a speed of

46

miles per hour. How long will it be before they meet? If necessary, round your answer to the nearest minute.

\_\_\_\_

\_\_\_\_

Posted 2 months ago

Question

\newline

500

=

Posted 3 months ago

Question

\newline

2,000

=

Posted 3 months ago

Question

\newline

750

=

Posted 3 months ago

Question

\newline

1,500

=

Posted 3 months ago

Question

\newline

1,500

=

Posted 3 months ago