Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the derivative of the following function.

y=4^(9x^(4))
Answer:
y^(')=

Find the derivative of the following function. $\newline$ $y=4^{9 x^{4}}$ $\newline$ Answer: $y^{\prime}=$

View step-by-step help

View step-by-step help

Find derivatives using logarithmic differentiation

Full solution

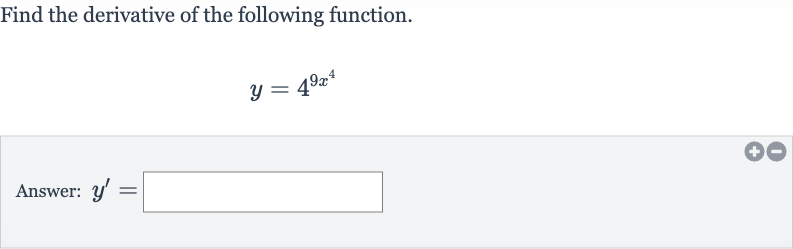
Q. Find the derivative of the following function.

\newline

y=4^{9 x^{4}}

\newline

Answer:

y^{\prime}=

Identify Function & Differentiation Type: Identify the function and the type of differentiation required. $\newline$ We are given the function $y=4^{9x^{4}}$ and we need to find its derivative with respect to $x$ . This is an example of a composite function where we have an exponential function with a base of $4$ and an exponent that is itself a function of $x$ . To differentiate this, we will use the chain rule.
Apply Chain Rule: Apply the chain rule for differentiation. $\newline$ The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. In this case, the outer function is $4^u$ where $u=9x^{4}$ , and the inner function is $u=9x^{4}$ . We will first differentiate the outer function with respect to $u$ , and then multiply it by the derivative of the inner function with respect to $x$ .
Differentiate Outer Function: Differentiate the outer function with respect to $u$ . The derivative of $4^u$ with respect to $u$ is $(4^u \cdot \ln(4))$ . This is because the derivative of $a^u$ with respect to $u$ is $a^u \cdot \ln(a)$ , where $\ln$ denotes the natural logarithm.
Differentiate Inner Function: Differentiate the inner function with respect to $x$ . The derivative of $9x^{4}$ with respect to $x$ is $36x^{3}$ . This is because the derivative of $x^n$ with respect to $x$ is $n\cdot x^{(n-1)}$ .
Combine Derivatives: Combine the derivatives using the chain rule. $\newline$ Now we multiply the derivative of the outer function by the derivative of the inner function to get the overall derivative of $y$ with respect to $x$ . $\newline$ $y' = (4^{9x^{4}} \cdot \ln(4)) \cdot 36x^{3}$
Simplify Expression: Simplify the expression if possible. $\newline$ In this case, there is no further simplification that can be done without changing the form of the expression. So, the derivative of the function $y=4^{9x^{4}}$ with respect to $x$ is $y' = (4^{9x^{4}} \cdot \ln(4)) \cdot 36x^{3}$ .

More problems from Find derivatives using logarithmic differentiation

Question

\newline

\sqrt{\frac{9}{25}}

\newline

Posted 2 months ago

Question

\newline

1,000

\text{ milligrams}

\_\_\_\_

\text{ grams}

Posted 2 months ago

Question

3

-gallon bottle of bleach costs

\$11.52

. What is the price per quart?

\newline

Posted 2 months ago

Question

Maureen can't wait to make chocolate chip zucchini bread with zucchini from her garden. Her recipe calls for

8

ounces of chocolate chips per loaf. If she has enough zucchini to make

4

loaves of zucchini bread, how many pounds of chocolate chips does she need?

\newline

Hint: There are

16

1

\newline

Write your answer as a whole number, decimal, or simplified fraction. Do not round.

\newline

Posted 2 months ago

Question

A whale is swimming due north at a speed of

30

miles per hour. Just

5

miles away, a whale-watching tour boat is traveling south, directly toward the whale, at a speed of

46

miles per hour. How long will it be before they meet? If necessary, round your answer to the nearest minute.

\_\_\_\_

\_\_\_\_

Posted 2 months ago

Question

\newline

500

=

Posted 3 months ago

Question

\newline

2,000

=

Posted 3 months ago

Question

\newline

750

=

Posted 3 months ago

Question

\newline

1,500

=

Posted 3 months ago

Question

\newline

1,500

=

Posted 3 months ago