Full solution

Q. Find

\lim _{h \rightarrow 0} \frac{3 \ln (e+h)-3 \ln (e)}{h}

\newline

Choose

1

answer:

\newline

(A)

\frac{1}{e}

\newline

(B)

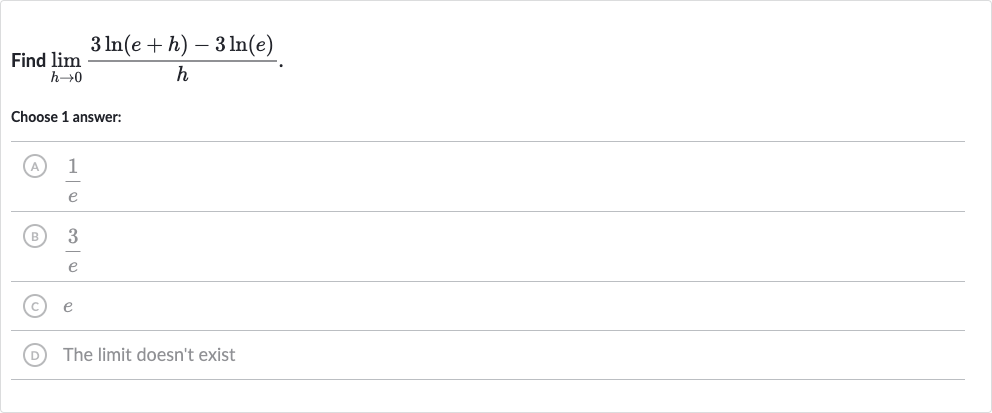
\frac{3}{e}

\newline

(c)

e

\newline

(D) The limit doesn't exist

Recognize base and function: Recognize that the limit involves the natural logarithm function and the constant $e$ , which is the base of the natural logarithm.
Rewrite using logarithm properties: Rewrite the expression inside the limit using the properties of logarithms: $\ln(a) - \ln(b) = \ln\left(\frac{a}{b}\right)$ .
Apply property to expression: Apply the property to the expression: $(3\ln(e+h)-3\ln(e))/h = 3\ln((e+h)/e)/h$ .
Simplify expression inside ln: Simplify the expression inside the ln function: $(e+h)/e = 1 + h/e$ .
Apply L'Hôpital's Rule: Now the expression is $3\ln(1 + \frac{h}{e})/h$ .
Derivative of numerator: Recognize that this is a standard limit form that can be solved using L'Hôpital's Rule, since it's in the $\frac{0}{0}$ indeterminate form as $h$ approaches $0$ .
Derivative of denominator: Apply L'Hôpital's Rule by taking the derivative of the numerator and the derivative of the denominator with respect to $h$ .
Simplify using derivatives: The derivative of the numerator $3\ln(1 + \frac{h}{e})$ with respect to $h$ is $\frac{3}{1 + \frac{h}{e}} \cdot \frac{1}{e}$ by the chain rule.
Take limit as $h$ approaches $0$ : The derivative of the denominator $h$ with respect to $h$ is $1$ .
Take limit as $h$ approaches $0$ : The derivative of the denominator $h$ with respect to $h$ is $1$ .Now the limit is $\frac{3}{(1 + \frac{h}{e})} \times \frac{1}{e} / 1$ , which simplifies to $\frac{3}{e + h}$ .
Take limit as $h$ approaches $0$ : The derivative of the denominator $h$ with respect to $h$ is $1$ .Now the limit is $\frac{3}{(1 + \frac{h}{e})} \cdot \frac{1}{e} / 1$ , which simplifies to $\frac{3}{e + h}$ .Take the limit as $h$ approaches $0$ , the expression becomes $\frac{3}{e}$ .