AI tutor
Full solution
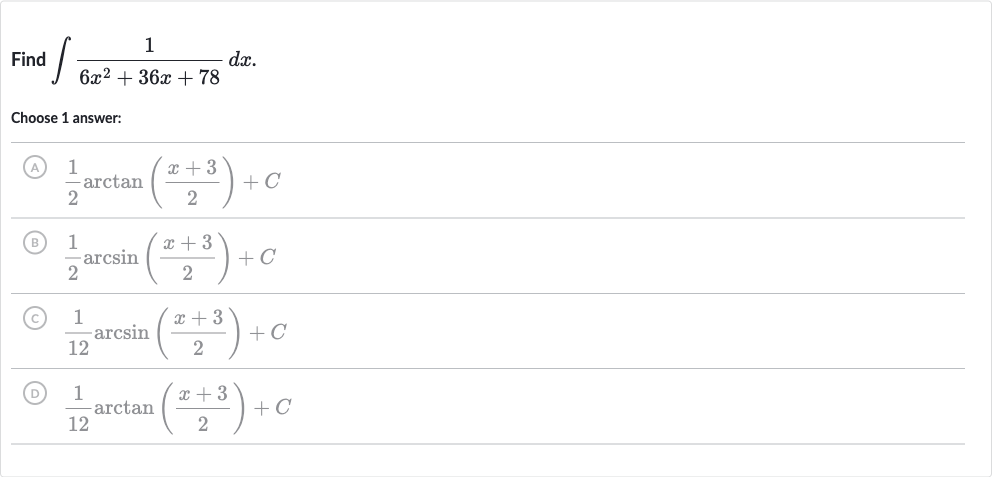
Q. Find .Choose answer:(A) (B) (C) (D)
- Complete the Square: First, complete the square for the quadratic in the denominator.Now, complete the square for .So,
- Factor Out and Simplify: Next, factor out the from the denominator to simplify the integral.
- Make Substitution: Now, let's make a substitution to make the integral look like the standard arctan integral form.Let , then .The integral becomes .
- Apply Arctan Integral Formula: The integral of is . Here, , so . The integral becomes .
- Substitute Back: Substitute back for to get the integral in terms of ..
- Check Answer Choices: Check the answer choices to see which one matches our result.The correct answer is (D) .