AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
Could , and be the side lengths of a triangle?Choose answer:(A) Yes(B) No
Full solution
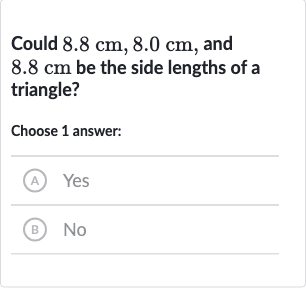
Q. Could , and be the side lengths of a triangle?Choose answer:(A) Yes(B) No
- Check Triangle Inequality Theorem: To determine if three lengths can form a triangle, we use the Triangle Inequality Theorem, which states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. We will check this for all three combinations of sides.
- Check Shorter Sides vs Longest Side: First, we check if the sum of the two shorter sides is greater than the longest side. The two shorter sides are and , and the longest side is . We calculate 8.0 + 8.0 > 8.8.
- Check Other Combinations: After performing the calculation, we find that 16.0 > 8.8, which is true. The first condition of the Triangle Inequality Theorem is satisfied.
- Check Third Combination: Next, we check the sum of the other two combinations. Since the sides are , , and , and we have already checked one combination, the other two combinations will be the same due to the two sides of being equal. We calculate 8.8 + 8.0 > 8.8.
- Verify Triangle Formation: After performing the calculation, we find that 16.8 > 8.8, which is true. The second condition of the Triangle Inequality Theorem is satisfied.
- Verify Triangle Formation: After performing the calculation, we find that 16.8 > 8.8, which is true. The second condition of the Triangle Inequality Theorem is satisfied. Finally, we check the third combination, which is the same as the second one due to the sides being equal. We calculate 8.8 + 8.0 > 8.8 again.
- Verify Triangle Formation: After performing the calculation, we find that 16.8 > 8.8, which is true. The second condition of the Triangle Inequality Theorem is satisfied. Finally, we check the third combination, which is the same as the second one due to the sides being equal. We calculate 8.8 + 8.0 > 8.8 again. After performing the calculation, we find that 16.8 > 8.8, which is true. The third condition of the Triangle Inequality Theorem is satisfied.
- Verify Triangle Formation: After performing the calculation, we find that 16.8 > 8.8, which is true. The second condition of the Triangle Inequality Theorem is satisfied. Finally, we check the third combination, which is the same as the second one due to the sides being equal. We calculate 8.8 + 8.0 > 8.8 again. After performing the calculation, we find that 16.8 > 8.8, which is true. The third condition of the Triangle Inequality Theorem is satisfied. Since all three conditions of the Triangle Inequality Theorem are satisfied, the given lengths can indeed form a triangle.
More problems from Is (x, y) a solution to the system of equations?
QuestionGet tutor help
