Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Could
13.5cm,8.0cm, and
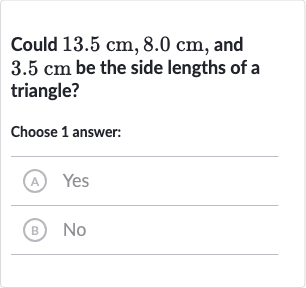
3.5cm be the side lengths of a triangle?
Choose 1 answer:
(A) Yes
(B) No

Could $13.5 \mathrm{~cm}, 8.0 \mathrm{~cm}$ , and $3.5 \mathrm{~cm}$ be the side lengths of a triangle? $\newline$ Choose $1$ answer: $\newline$ (A) Yes $\newline$ (B) No

View step-by-step help

View step-by-step help

Is (x, y) a solution to the system of equations?

Full solution

Q. Could

13.5 \mathrm{~cm}, 8.0 \mathrm{~cm}

, and

3.5 \mathrm{~cm}

be the side lengths of a triangle?

\newline

Choose

1

answer:

\newline

(A) Yes

\newline

(B) No

Use Triangle Inequality Theorem: To determine if three lengths can form a triangle, we can use the Triangle Inequality Theorem, which states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
Check Shortest Sides: First, we check if the sum of the two shortest sides is greater than the longest side. The two shortest sides are $8.0\,\text{cm}$ and $3.5\,\text{cm}$ , and the longest side is $13.5\,\text{cm}$ . We calculate $8.0\,\text{cm} + 3.5\,\text{cm}$ and compare it to $13.5\,\text{cm}$ .
Calculate Sum: The sum of the two shortest sides is $8.0\,\text{cm} + 3.5\,\text{cm} = 11.5\,\text{cm}$ . This sum must be greater than the longest side, which is $13.5\,\text{cm}$ , to satisfy the Triangle Inequality Theorem.
Verify Triangle Formation: Since $11.5\,\text{cm}$ is not greater than $13.5\,\text{cm}$ , the lengths $13.5\,\text{cm}$ , $8.0\,\text{cm}$ , and $3.5\,\text{cm}$ do not satisfy the Triangle Inequality Theorem and therefore cannot form a triangle.

More problems from Is (x, y) a solution to the system of equations?

Question

At the beginning of the year, Adrian's savings account balance was

\$ 28

. Each week, he deposits another

\$ 15

into that account, and he doesn't spend any of his savings.

\newline

Is his savings account balance proportional to the number of weeks since the start of the year?

\newline

1

\newline

\newline

Posted 3 months ago

Question

Anna does sit-ups to get ready for her first triathlon. When she starts, she does a sit-up every

2

seconds. But, as she gets tired, each sit-up takes longer and longer to do.

\newline

Is the number of sit-ups Anna does proportional to the time she spends doing them?

\newline

1

\newline

\newline

Posted 3 months ago

Question

Today, Howell has

75

kiwis. He will eat

2

of them every day.

\newline

Is the number of kiwis Howell has left proportional to the number of days that pass?

\newline

1

\newline

\newline

Posted 3 months ago

Question

y=-8 x-3

\newline

x+y=7

\newline

(3,4)

a solution of the system?

\newline

1

\newline

\newline

Posted 3 months ago

Question

What is the result of subtracting the second equation from the first?

\newline

-2 x+y=0

\newline

-7 x+3 y=2

\newline

\square

Posted 3 months ago

Question

What is the result of subtracting the second equation from the first?

\newline

\begin{array}{l} 2 x+7 y=-8 \\ 2 x-5 y=-1 \end{array}

\newline

\square

Posted 3 months ago

Question

What is the result of adding these two equations?

\newline

5 x-y=6

\newline

-2 x+y=8

\newline

\square

Posted 3 months ago

Question

10.7 \mathrm{~cm}, 3.2 \mathrm{~cm}

5.5 \mathrm{~cm}

be the side lengths of a triangle?

\newline

1

\newline

\newline

Posted 3 months ago

Question

7.7 \mathrm{~cm}, 4.0 \mathrm{~cm}

1.7 \mathrm{~cm}

be the side lengths of a triangle?

\newline

1

\newline

\newline

Posted 3 months ago

Question

Isabel tried to evaluate an expression step by step.

(2+8)+8

. Find Isabel's first mistake

Posted 13 hours ago