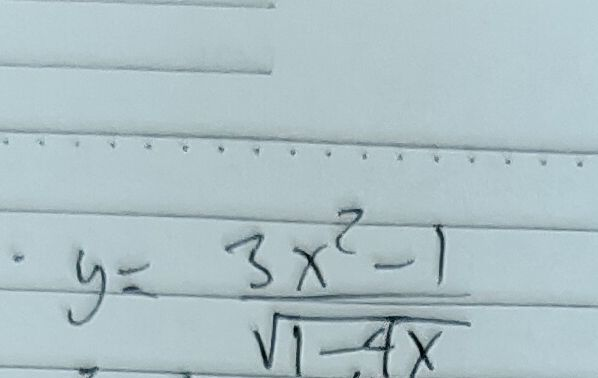y=(3x^(2)-1)/(sqrt(1-4x))

Question

Bytelearn · Accepted Answer

Identify Components: First, we need to identify the components of the expression. We have a numerator which is a polynomial (3x^2 - 1) and a denominator which is a square root of a binomial (sqrt(1 - 4x)). There is no immediate simplification that can be done since the numerator and the denominator are not similar terms and cannot be factored in a way that would cancel out terms across the numerator and denominator.Check Domain Restriction: Next, we should check if there are any restrictions on the domain of the function due to the square root in the denominator. The expression inside the square root, (1 - 4x), must be greater than or equal to zero because the square root of a negative number is not defined in the set of real numbers. Therefore, we have the inequality 1 - 4x >= 0, which simplifies to x <= 1/4. This is a domain restriction, not a simplification of the expression.Expression Simplification: Since there are no common factors between the numerator and the denominator, and no further algebraic simplification is possible, the expression y=(3x^2-1)/(sqrt(1-4x)) is already in its simplest form. We cannot simplify it further without knowing the specific value of x (which must satisfy the domain restriction x <= 1/4).

$y=\frac{3 x^{2}-1}{\sqrt{1-4 x}}$

Full solution

More problems from Simplify variable expressions using properties

Related topics

y=3x2−11−4x y=\frac{3 x^{2}-1}{\sqrt{1-4 x}} y=1−4x​3x2−1​

$y=\frac{3 x^{2}-1}{\sqrt{1-4 x}}$