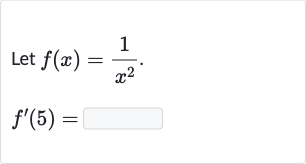AI tutor
Full solution
Q. Let .
- Apply Power Rule: To find the derivative of the function , we need to apply the power rule for derivatives. The power rule states that the derivative of with respect to is . In this case, we can rewrite the function as and then apply the power rule.
- Calculate Derivative: Applying the power rule, we get . This simplifies the derivative of the function to .
- Substitute : Now we need to evaluate the derivative at . We substitute with in the derivative function to get .
- Evaluate : Calculating the value of , we have .
More problems from Simplify variable expressions using properties
QuestionGet tutor help