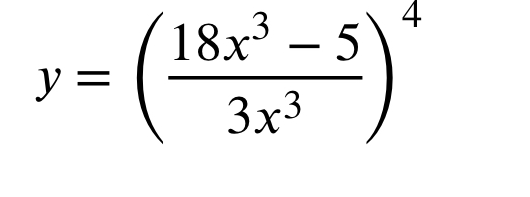AI tutor
Full solution
Q.
- Simplify Function: First, simplify the function before taking the derivative.Simplify the fraction inside the parentheses by dividing both the numerator and the denominator by .Now the function is simplified.
- Apply Chain Rule: Next, apply the chain rule to find the derivative of with respect to . The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function.Let , then .First, find the derivative of with respect to , which is .
- Find with respect to : Now, find the derivative of with respect to , .
- Apply Chain Rule: Now, apply the chain rule by multiplying by to get .
Substitute back into the equation. - Simplify Derivative: Finally, simplify the derivative expression.This is the derivative of the function with respect to .