Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

x=78(7.7-t)
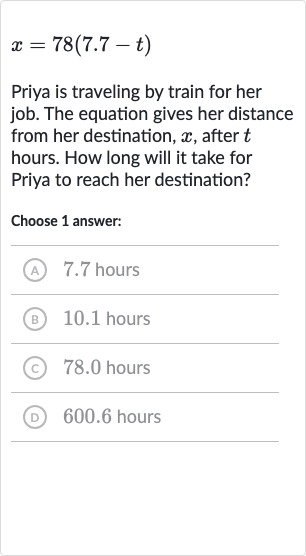
Priya is traveling by train for her job. The equation gives her distance from her destination,
x, after
t hours. How long will it take for Priya to reach her destination?
Choose 1 answer:
(A) 7.7 hours
(B) 10.1 hours
(c) 78.0 hours
(D) 600.6 hours

$x=78(7.7-t)$ $\newline$ Priya is traveling by train for her job. The equation gives her distance from her destination, $x$ , after $t$ hours. How long will it take for Priya to reach her destination? $\newline$ Choose $1$ answer: $\newline$ (A) $7$ . $7$ hours $\newline$ (B) $\mathbf{1 0 . 1}$ hours $\newline$ (C) $78$ . $0$ hours $\newline$ (D) $600$ . $6$ hours

View step-by-step help

View step-by-step help

Multi-step problems with unit conversions

Full solution

Q.

x=78(7.7-t)

\newline

Priya is traveling by train for her job. The equation gives her distance from her destination,

x

, after

t

hours. How long will it take for Priya to reach her destination?

\newline

Choose

1

answer:

\newline

(A)

7

.

7

hours

\newline

(B)

\mathbf{1 0 . 1}

hours

\newline

(C)

78

.

0

hours

\newline

(D)

600

.

6

hours

Given Equation: We are given the equation $x = 78(7.7 - t)$ which represents Priya's distance from her destination after $t$ hours. To find out how long it will take for Priya to reach her destination, we need to find the value of $t$ when $x$ equals $0$ , because reaching the destination means no distance left to travel.
Set Equal to Zero: Set the equation equal to zero to find when Priya reaches her destination: $0 = 78(7.7 - t)$ .
Divide by $78$ : Divide both sides of the equation by $78$ to isolate the term in parentheses: $\frac{0}{78} = (7.7 - t)$ .
Simplify Equation: Simplify the left side of the equation, knowing that $0$ divided by any number is $0$ : $0 = 7.7 - t$ .
Subtract $7$ . $7$ : To solve for $t$ , we need to get $t$ on one side of the equation by itself. We can do this by subtracting $7.7$ from both sides of the equation: $-7.7 = -t$ .
Multiply by $-1$ : Now, we multiply both sides of the equation by $-1$ to solve for $t$ : $t = 7.7$ .

More problems from Multi-step problems with unit conversions

Question

\newline

1,000

\text{ milligrams}

\_\_\_\_

\text{ grams}

Posted 2 months ago

Question

3

-gallon bottle of bleach costs

\$11.52

. What is the price per quart?

\newline

Posted 2 months ago

Question

Maureen can't wait to make chocolate chip zucchini bread with zucchini from her garden. Her recipe calls for

8

ounces of chocolate chips per loaf. If she has enough zucchini to make

4

loaves of zucchini bread, how many pounds of chocolate chips does she need?

\newline

Hint: There are

16

1

\newline

Write your answer as a whole number, decimal, or simplified fraction. Do not round.

\newline

Posted 2 months ago

Question

A whale is swimming due north at a speed of

30

miles per hour. Just

5

miles away, a whale-watching tour boat is traveling south, directly toward the whale, at a speed of

46

miles per hour. How long will it be before they meet? If necessary, round your answer to the nearest minute.

\_\_\_\_

\_\_\_\_

Posted 2 months ago

Question

\newline

500

=

Posted 3 months ago

Question

\newline

2,000

=

Posted 3 months ago

Question

\newline

750

=

Posted 3 months ago

Question

\newline

1,500

=

Posted 3 months ago

Question

\newline

1,500

=

Posted 3 months ago

Question

The formula for the time it takes to travel a distance of

d

at an average speed

v

\frac{d}{v}

100

-meter dash at a speed of about

7.5

meters per second. What calculation will give us the estimated duration of Tessa's dash in seconds? Choose

1

\newline

\frac{7.5}{100}

\newline

\frac{100}{7.5}

\newline

\frac{7.5}{100 \times 60}

\newline

\frac{100 \times 60}{7.5}

Posted 4 months ago