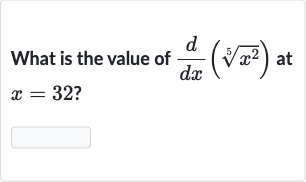AI tutor
Full solution
Q. What is the value of at ?
- Rewrite function: We need to find the derivative of the function . To do this, we will use the power rule for differentiation, which states that if , then .
- Apply power rule: First, we rewrite the fifth root of squared as to make it easier to differentiate.
- Find derivative: Now we apply the power rule for differentiation to find .
- Evaluate at : Next, we need to evaluate the derivative at .
- Simplify expression: We simplify the expression by calculating . Since is , we can rewrite as .
- Calculate value: Now we calculate , which is .
- Calculate value: Now we calculate , which is . Finally, we multiply by to get the value of the derivative at .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help