AI tutor
Full solution
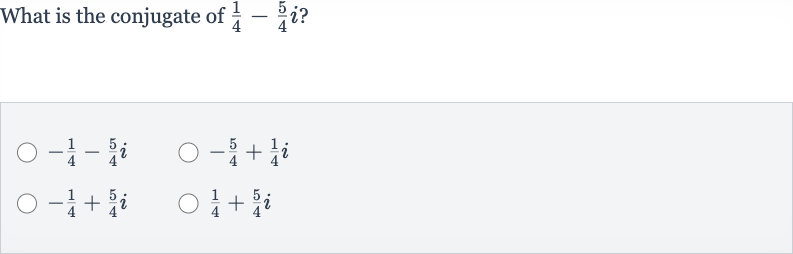
Q. What is the conjugate of ?
- Find Conjugate: The conjugate of a complex number is . To find the conjugate, we simply change the sign of the imaginary part.
- Given Complex Number: Given the complex number , the conjugate will be , because we change the sign of the imaginary part from negative to positive.
- Check Options: Check the given options to find the correct conjugate.
- Correct Conjugate: The correct conjugate of is , which matches one of the given options.
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help