Full solution
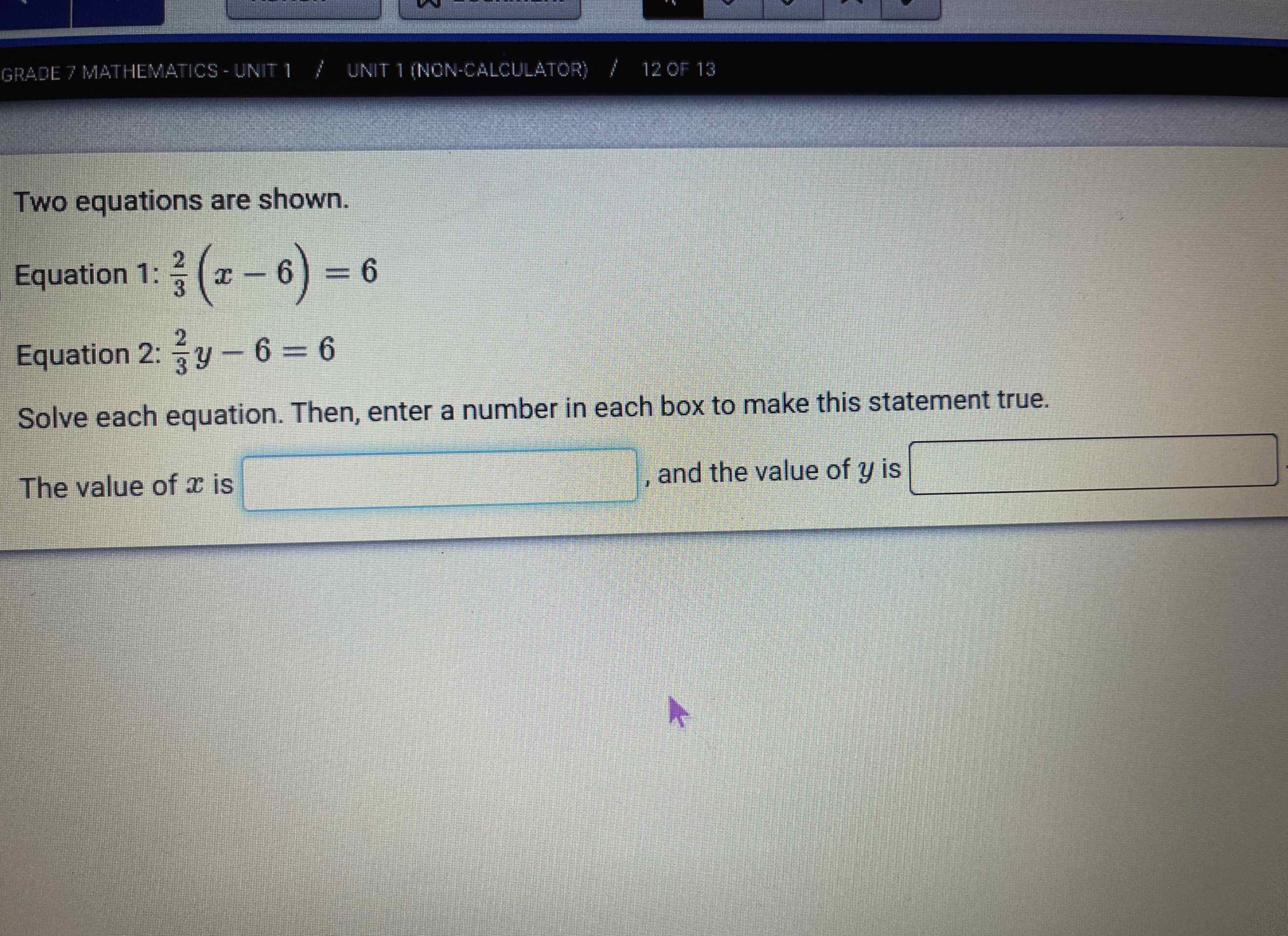
Q. Two equations are shown. Equation : Equation : Solve each equation. Then, enter a number in each box to make this statement true. The value of x is ◻ , and the value of y is ◻
- Solve for x: Step : Solve Equation for x.Equation is .First, isolate by multiplying both sides by .
- Find : Step : Now, solve for . Add to both sides of the equation.
- Solve for y: Step : Solve Equation for y.Equation is .First, add to both sides to isolate .
- Find y: Step : Now, solve for y.Multiply both sides by to isolate y.y =
More problems from Find a value using two-variable equations: word problems
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help