Full solution
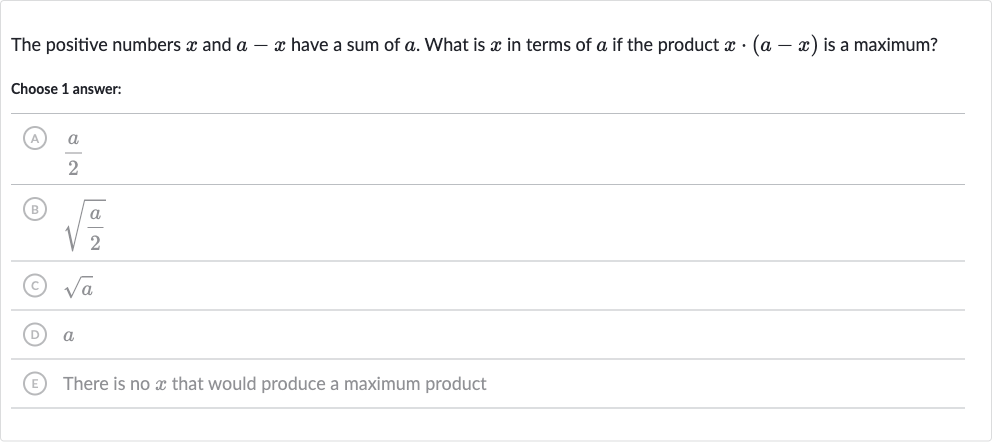
Q. The positive numbers and have a sum of . What is in terms of if the product is a maximum?Choose answer:(A) (B) (C) (D) (E) There is no that would produce a maximum product
- Equation Simplification: We know that . This simplifies to , which is always true, so we don't get information about from this equation.
- Maximizing Product: To maximize the product , we can use the idea that for a fixed sum, the product of two numbers is maximized when the numbers are equal. So, we set .
- Solving for : Solving gives us , so .
- Final Answer: Therefore, the value of that maximizes the product is , which corresponds to answer choice (A).
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help