The height of a river above a fixed point on the riverbed was monitored over a 7-day period. The height of the river, H metres, t days after monitoring began, was given by H=(sqrtt)/(20)(20+6t-t^(2))+17quad0

Question

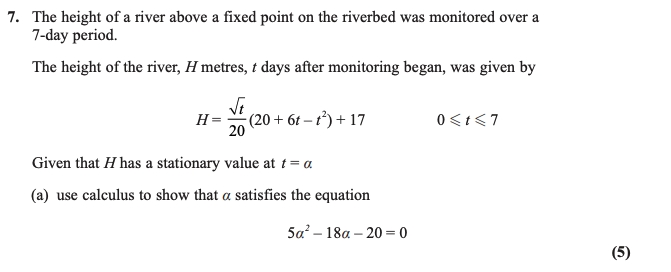
The height of a river above a fixed point on the riverbed was monitored over a 7-day period. The height of the river,  H metres,  t days after monitoring began, was given by   H=(sqrtt)/(20)(20+6t-t^(2))+17quad0 <= t <= 7 Given that  H has a stationary value at  t=alpha (a) use calculus to show that  alpha satisfies the equation  5alpha^(2)-18 alpha-20=0

Bytelearn · Accepted Answer

Differentiate with Quotient Rule: To find the stationary value of H, we need to differentiate H with respect to t and set the derivative equal to zero.Calculate Derivatives: Differentiate H with respect to t using the quotient rule: (d/dt)[(sqrt(t))/(20)(20+6t-t^2)].Apply Quotient Rule Formula: Let u = sqrt(t) and v = (20)(20+6t-t^2). Then du/dt = 1/(2sqrt(t)) and dv/dt = 6 - 2t.Simplify Derivative: Using the quotient rule, dH/dt = (v(du/dt) - u(dv/dt))/v^2.Set Derivative Equal to Zero: Substitute u, du/dt, v, and dv/dt into the quotient rule formula: dH/dt = ((20)(20+6t-t^2)(1/(2sqrt(t))) - (sqrt(t))(6 - 2t))/((20)(20+6t-t^2))^2.Clear Denominators: Simplify the derivative: dH/dt = (400 + 120t - 20t^2)/(40sqrt(t)) - (6sqrt(t) - 2tsqrt(t))/(400 + 120t - 20t^2).Final Equation: To find the stationary points, set dH/dt equal to zero: (400 + 120t - 20t^2)/(40sqrt(t)) - (6sqrt(t) - 2tsqrt(t))/(400 + 120t - 20t^2) = 0.Multiply both sides by (40sqrt(t))(400 + 120t - 20t^2) to clear the denominators.After multiplying, we get: (400 + 120t - 20t^2) - (6t - 2t^2)(40sqrt(t)) = 0.

Full solution

More problems from Find derivatives of sine and cosine functions

Related topics