Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

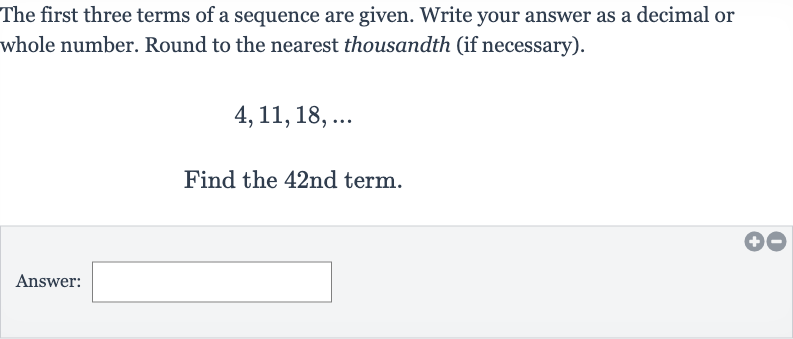
The first three terms of a sequence are given. Write your answer as a decimal or whole number. Round to the nearest thousandth (if necessary).

4,11,18,dots
Find the 42nd term.
Answer:

The first three terms of a sequence are given. Write your answer as a decimal or whole number. Round to the nearest thousandth (if necessary). $\newline$ $4,11,18, \ldots$ $\newline$ Find the $42$ nd term. $\newline$ Answer:

View step-by-step help

View step-by-step help

Powers with decimal bases

Full solution

Q. The first three terms of a sequence are given. Write your answer as a decimal or whole number. Round to the nearest thousandth (if necessary).

\newline

4,11,18, \ldots

\newline

Find the

42

nd term.

\newline

Answer:

Identify pattern: Identify the pattern in the sequence. $\newline$ The sequence starts with $4$ and each term increases by $7$ ( $11 - 4 = 7$ , $18 - 11 = 7$ ). $\newline$ This is an arithmetic sequence with a common difference of $7$ .
Use formula: Use the formula for the $n$ th term of an arithmetic sequence. $\newline$ The $n$ th term ( $a_n$ ) of an arithmetic sequence can be found using the formula: $\newline$ $a_n = a_1 + (n - 1)d$ $\newline$ where $a_1$ is the first term, $n$ is the term number, and $d$ is the common difference.
Plug values: Plug in the values for the $42^{\text{nd}}$ term. $a_1 = 4$ (the first term) $n = 42$ (the term number we want to find) $d = 7$ (the common difference) $a_{42} = 4 + (42 - 1) \times 7$
Perform calculation: Perform the calculation. $\newline$ $a_{42} = 4 + (41) \times 7$ $\newline$ $a_{42} = 4 + 287$ $\newline$ $a_{42} = 291$

More problems from Powers with decimal bases

Question

Write the expression using an exponent.

\newline

2 \times 2

\newline

Posted 3 months ago

Question

Evaluate the expression.

4 - 4^2

Posted 3 months ago

Question

x^2 = 4

Posted 2 months ago

Question

x^2 = 9

Posted 4 months ago

Question

\newline

x^2 = 0

\newline

Write your answer in simplified, rationalized form.

\newline

x =

x =

Posted 4 months ago

Question

x

\newline

8 = 2^x

\newline

x =

Posted 4 months ago

Question

x

\newline

6 = 7^x

\newline

x =

Posted 4 months ago

Question

Select the equivalent expression.

\newline

((8^{-5})/(2^{-2}))^{-4}=?

\newline

1

\newline

\newline

(8^{20})/(2^{8})

\newline

\newline

(2^{6})/(8^{9})

\newline

\newline

(1)/(8\cdot 2^{2})

Posted 16 days ago

Question

Select the equivalent expression.

\newline

\left(\frac{b^{7}}{4^{5}}\right)^{-3}=\,?

\newline

1

\newline

\frac{b^{21}}{4^{15}}

\newline

\frac{4^{15}}{b^{21}}

\newline

b^{-21}\cdot 4^{-15}

Posted 2 months ago

Question

Select the equivalent expression.

\newline

(3^{3}\cdot6^{6})^{-3}=

\newline

1

\newline

\frac{1}{3^{9}\cdot6^{18}}

\newline

\frac{6^{18}}{3^{9}}

\newline

\frac{3^{9}}{6^{18}}

Posted 2 months ago