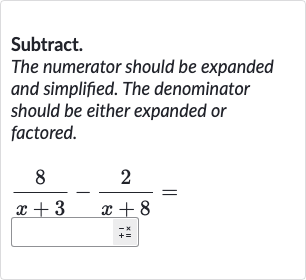
Subtract. The numerator should be expanded and simplified. The denominator should be either expanded or factored. (8)/(x+3)-(2)/(x+8)=

Question

Subtract. The numerator should be expanded and simplified. The denominator should be either expanded or factored.  (8)/(x+3)-(2)/(x+8)=

Bytelearn · Accepted Answer

Identify common denominator: Identify the common denominator for the two fractions. To subtract the fractions, we need a common denominator. The common denominator for (x+3) and (x+8) is the product of the two, which is (x+3)(x+8).Rewrite fractions with common denominator: Rewrite each fraction with the common denominator. We need to express each fraction with the common denominator (x+3)(x+8). This means multiplying the numerator and denominator of each fraction by the necessary factor to achieve the common denominator. For the first fraction, (8)/(x+3), we multiply the numerator and denominator by (x+8). For the second fraction, (2)/(x+8), we multiply the numerator and denominator by (x+3).Perform multiplication for each fraction: Perform the multiplication for each fraction. Now we multiply the numerators and denominators as determined in Step 2. First fraction: (8 * (x+8)) / ((x+3)(x+8)) Second fraction: (2 * (x+3)) / ((x+3)(x+8))Expand numerators: Expand the numerators. We need to expand the numerators of both fractions. First fraction's numerator: 8 * (x+8) = 8x + 64 Second fraction's numerator: 2 * (x+3) = 2x + 6Combine fractions by subtracting numerators: Combine the fractions by subtracting the numerators. Now that we have a common denominator, we can combine the fractions by subtracting the second fraction's numerator from the first fraction's numerator. (8x + 64) - (2x + 6) = 8x + 64 - 2x - 6Simplify the result: Simplify the result. Simplify the expression by combining like terms. 8x + 64 - 2x - 6 = (8x - 2x) + (64 - 6) = 6x + 58Write the final answer: Write the final answer. The final answer is the simplified numerator over the common denominator. (6x + 58) / ((x+3)(x+8))

Subtract. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{8}{x+3}-\frac{2}{x+8}=$

Full solution

More problems from Negative Exponents

Related topics

Subtract.\newlineThe numerator should be expanded and simplified. The denominator should be either expanded or factored.\newline8x+3−2x+8= \frac{8}{x+3}-\frac{2}{x+8}= x+38​−x+82​=

Subtract. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{8}{x+3}-\frac{2}{x+8}=$