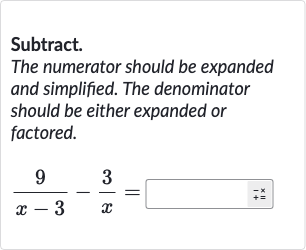
Subtract. The numerator should be expanded and simplified. The denominator should be either expanded or factored. (9)/(x-3)-(3)/(x)=◻

Question

Subtract. The numerator should be expanded and simplified. The denominator should be either expanded or factored.  (9)/(x-3)-(3)/(x)=◻

Bytelearn · Accepted Answer

Identify common denominator: Identify the common denominator for the two fractions. To subtract the fractions (9)/(x-3) and (3)/(x), we need a common denominator. The common denominator will be the product of the two distinct denominators, which are (x-3) and x. Common Denominator: (x-3)*xRewrite fractions with common denominator: Rewrite each fraction with the common denominator. We need to adjust each fraction so that they both have the common denominator. This means multiplying the numerator and denominator of each fraction by the term that is missing from its current denominator. (9)/(x-3) becomes (9*x)/(x*(x-3)) (3)/(x) becomes (3*(x-3))/(x*(x-3))Expand numerators of new fractions: Expand the numerators of the new fractions. Now we expand the numerators of both fractions to simplify the subtraction. (9*x)/(x*(x-3)) becomes (9x)/(x*(x-3)) (3*(x-3))/(x*(x-3)) becomes (3x - 9)/(x*(x-3))Subtract second fraction from first fraction: Subtract the second fraction from the first fraction. Now that both fractions have the same denominator, we can subtract the numerators directly. (9x)/(x*(x-3)) - (3x - 9)/(x*(x-3)) = (9x - (3x - 9))/(x*(x-3))Simplify numerator of resulting fraction: Simplify the numerator of the resulting fraction. We need to distribute the negative sign in the numerator and combine like terms. (9x - (3x - 9)) = 9x - 3x + 9 = 6x + 9 So the simplified numerator is 6x + 9.Write final simplified fraction: Write the final simplified fraction. The final simplified fraction with the common denominator and simplified numerator is: (6x + 9)/(x*(x-3))

Subtract. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{9}{x-3}-\frac{3}{x}=\square$

Full solution

More problems from Negative Exponents

Related topics

Subtract.\newlineThe numerator should be expanded and simplified. The denominator should be either expanded or factored.\newline9x−3−3x=□ \frac{9}{x-3}-\frac{3}{x}=\square x−39​−x3​=□

Subtract. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{9}{x-3}-\frac{3}{x}=\square$