AI tutor
Full solution
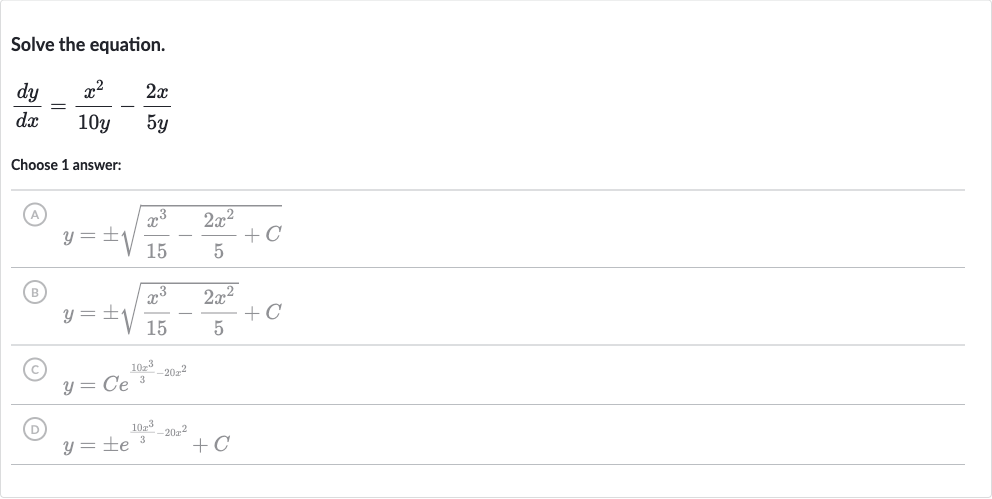
Q. Solve the equation.Choose answer:(A) (B) (C) (D)
- Combine terms over common denominator: We are given the differential equation:To solve this, we will first combine the terms on the right-hand side over a common denominator.
- Separate variables and multiply: Now, we will separate variables by multiplying both sides by and , and dividing by .
- Integrate both sides: Next, we integrate both sides of the equation.
- Solve for y: Now, we solve for y by taking the square root of both sides.