Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve for x. (2x+2)/(x+4)=(4)/(5) Answer:

Solve for x \mathrm{x} .\newline2x+2x+4=45 \frac{2 x+2}{x+4}=\frac{4}{5} \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 8right chevron icon
Negative Exponentsright chevron icon

Full solution

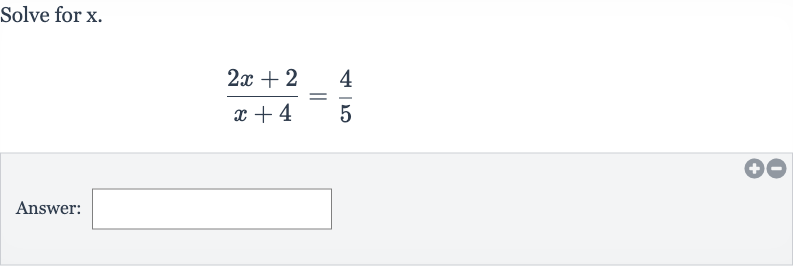
Q. Solve for x \mathrm{x} .\newline2x+2x+4=45 \frac{2 x+2}{x+4}=\frac{4}{5} \newlineAnswer:
  1. Write Equation: Write down the equation to be solved.\newlineWe are given the equation (2x+2)/(x+4)=4/5(2x+2)/(x+4) = 4/5. We need to find the value of xx that satisfies this equation.
  2. Cross-Multiply: Cross-multiply to eliminate the fractions.\newlineTo solve for xx, we can cross-multiply the equation to get rid of the fractions. This means we multiply the numerator of one fraction by the denominator of the other fraction and set it equal to the product of the remaining numerator and denominator.\newline(2x+2)×5=4×(x+4)(2x + 2) \times 5 = 4 \times (x + 4)
  3. Distribute Multiplication: Distribute the multiplication over addition on both sides of the equation.\newlineNow we distribute the multiplication over addition to simplify both sides of the equation.\newline(2x×5)+(2×5)=4x+(4×4)(2x \times 5) + (2 \times 5) = 4x + (4 \times 4)\newline10x+10=4x+1610x + 10 = 4x + 16
  4. Move Terms: Move all terms containing xx to one side and constant terms to the other side.\newlineWe want to isolate xx on one side of the equation, so we'll subtract 4x4x from both sides and subtract 1010 from both sides to move the constants to the other side.\newline10x+10−4x−10=4x+16−4x−1010x + 10 - 4x - 10 = 4x + 16 - 4x - 10\newline6x=66x = 6
  5. Divide Coefficient: Divide both sides of the equation by the coefficient of xx to solve for xx. To isolate xx, we divide both sides of the equation by 66. 6x6=66\frac{6x}{6} = \frac{6}{6} x=1x = 1

More problems from Negative Exponents

Question
Write the expression using an exponent.\newline2×22 \times 2\newline______
Get tutor helpright-arrow

Posted 8 months ago

Question
Evaluate the expression. 4−42 4 - 4^2 ____
Get tutor helpright-arrow

Posted 8 months ago

Question
x2=4x^2 = 4
Get tutor helpright-arrow

Posted 7 months ago

Question
x2=9x^2 = 9
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for x. \newlinex2=0x^2 = 0 \newlineWrite your answer in simplified, rationalized form. \newlinex=x = _____ or x=x = _____
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for xx. \newline8=2x8 = 2^x \newline x=x = _____
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for xx. \newline6=7x6 = 7^x \newline x=x = _____
Get tutor helpright-arrow

Posted 9 months ago

Question
Select the equivalent expression.\newline((8−5)/(2−2))−4=?((8^{-5})/(2^{-2}))^{-4}=?\newlineChoose 11 answer:\newline(A) \newline(820)/(28)(8^{20})/(2^{8})\newline(B) \newline(26)/(89)(2^{6})/(8^{9})\newline(C) \newline(1)/(8⋅22)(1)/(8\cdot 2^{2})
Get tutor helpright-arrow

Posted 6 months ago

Question
Select the equivalent expression.\newline(b745)−3= ?\left(\frac{b^{7}}{4^{5}}\right)^{-3}=\,?\newlineChoose 11 answer:\newline(A) b21415\frac{b^{21}}{4^{15}}\newline(B) 415b21\frac{4^{15}}{b^{21}}\newline(C) b−21⋅4−15b^{-21}\cdot 4^{-15}
Get tutor helpright-arrow

Posted 7 months ago

Question
Select the equivalent expression.\newline(33⋅66)−3=(3^{3}\cdot6^{6})^{-3}=?\newlineChoose 11 answer:\newline(A) 139⋅618\frac{1}{3^{9}\cdot6^{18}}\newline(B) 61839\frac{6^{18}}{3^{9}}\newline(C) 39618\frac{3^{9}}{6^{18}}
Get tutor helpright-arrow

Posted 8 months ago

View all (89)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant