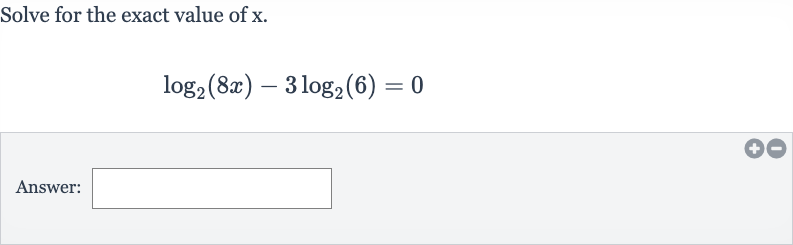
Solve for the exact value of x. log_(2)(8x)-3log_(2)(6)=0 Answer:

Question

Solve for the exact value of  x.  log_(2)(8x)-3log_(2)(6)=0 Answer:

Bytelearn · Accepted Answer

Apply power rule: Apply the power rule of logarithms to the term 3log₂(6). The power rule states that a*log_b(c) = log_b(c^a), where a is a constant, b is the base of the logarithm, and c is the argument of the logarithm. So, 3log₂(6) becomes log₂(6^3).Rewrite equation: Rewrite the equation using the result from Step 1. log₂(8x) - log₂(6^3) = 0Combine logarithms: Combine the logarithms on the left side of the equation using the quotient rule. The quotient rule states that log_b(m) - log_b(n) = log_b(m/n), where b is the base of the logarithm, m and n are the arguments of the logarithm. So, log₂(8x) - log₂(6^3) becomes log₂((8x)/(6^3)).Simplify argument: Simplify the argument of the logarithm. (8x)/(6^3) simplifies to (8x)/216.Set equal to 2^0: Set the argument of the logarithm equal to 2^0, since the right side of the equation is 0 and log₂(2^0) = 0. log₂((8x)/216) = log₂(2^0) This implies that (8x)/216 = 2^0.Solve for x: Solve for x. Since 2^0 = 1, we have (8x)/216 = 1. Multiplying both sides by 216 gives 8x = 216. Dividing both sides by 8 gives x = 216/8.Calculate exact value: Calculate the exact value of x. x = 216/8 x = 27

Solve for the exact value of $x$ . $\newline$ $\log _{2}(8 x)-3 \log _{2}(6)=0$ $\newline$ Answer:

Full solution

More problems from Find derivatives of logarithmic functions

Related topics

Solve for the exact value of x x x.\newlinelog⁡2(8x)−3log⁡2(6)=0 \log _{2}(8 x)-3 \log _{2}(6)=0 log2​(8x)−3log2​(6)=0\newlineAnswer:

Solve for the exact value of $x$ . $\newline$ $\log _{2}(8 x)-3 \log _{2}(6)=0$ $\newline$ Answer: