AI tutor
Full solution
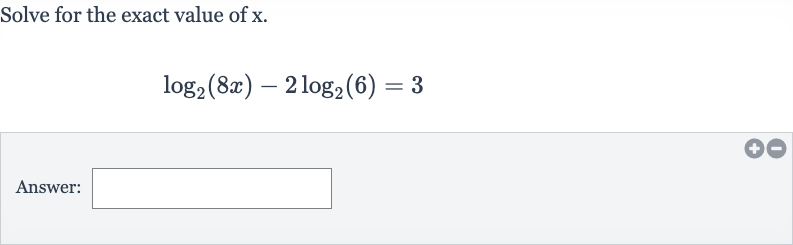
Q. Solve for the exact value of .Answer:
- Apply power rule of logarithms: Apply the power rule of logarithms to the term . The power rule states that . Therefore, we can rewrite as .
- Simplify term : Simplify the term . equals , so the equation becomes:
- Apply quotient rule of logarithms: Apply the quotient rule of logarithms to combine the logarithmic terms.The quotient rule states that . Therefore, we can combine the logarithmic terms:
- Convert to exponential equation: Convert the logarithmic equation to an exponential equation.Using the definition of a logarithm, we can rewrite the equation as:
- Calculate : Calculate . equals , so the equation becomes:
- Multiply by : Multiply both sides of the equation by to solve for .
- Divide by : Divide both sides of the equation by to isolate .