AI tutor
Full solution
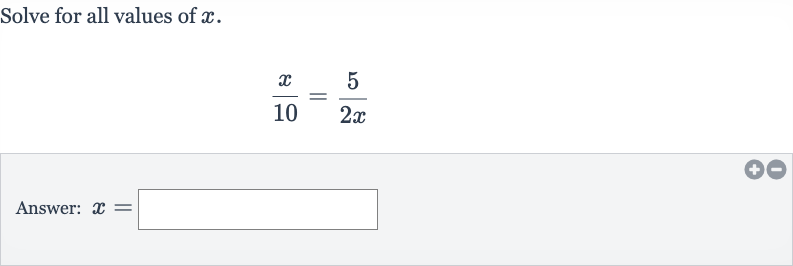
Q. Solve for all values of .Answer:
- Cross-multiply fractions: Cross-multiply to eliminate the fractions.We have . To remove the fractions, we multiply both sides by and , which are the denominators of the fractions.So,
- Simplify equation: Simplify the equation.Now we have .
- Divide to isolate : Divide both sides by to isolate .
- Take square root: Take the square root of both sides to solve for .
More problems from Powers with negative bases
QuestionGet tutor help