Resources

Testimonials

Plans

Resources

Testimonials

Plans

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

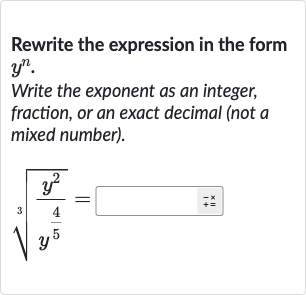
$Rewrite the expression in the form y^(n). Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). root(3)((y^(2))/(y^((4)/(5))))=◻$

Rewrite the expression in the form $y^{n}$ . $\newline$ Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). $\newline$ $\sqrt[3]{\frac{y^{2}}{y^{\frac{4}{5}}}}=\square$

View step-by-step help

Home

Math Problems

Algebra 1

Powers with decimal and fractional bases

Full solution

Q. Rewrite the expression in the form

y^{n}

\newline

Write the exponent as an integer, fraction, or an exact decimal (not a mixed number).

\newline

\sqrt[3]{\frac{y^{2}}{y^{\frac{4}{5}}}}=\square

Rewrite as Exponent: Apply the property of radicals to rewrite the cube root as an exponent. $\sqrt[3]{\frac{y^{2}}{y^{\frac{4}{5}}}} = \frac{y^{2^{\frac{1}{3}}}}{y^{\left(\frac{4}{5}\right)^{\frac{1}{3}}}}$
Simplify with Power Rule: Use the power rule of exponents to simplify the expression. $\newline$ $y^{(2)}$ ^{( $1$ / $3$ )} becomes $y^{(2*(1/3))}$ which simplifies to $y^{(2/3)}$ . $\newline$ $y^{(4/5)}$ ^{( $1$ / $3$ )} becomes $y^{((4/5)*(1/3))}$ which simplifies to $y^{(4/15)}$ .
Divide Using Quotient Rule: Now, divide the two expressions using the quotient rule for exponents. $y^{\frac{2}{3}} / y^{\frac{4}{15}}$
Subtract Exponents: To divide the expressions with the same base, subtract the exponents. $y^{\frac{2}{3} - \frac{4}{15}}$
Find Common Denominator: Find a common denominator to subtract the fractions in the exponents. $\newline$ The common denominator for $3$ and $15$ is $15$ . $\newline$ $y^{(\frac{10}{15} - \frac{4}{15})}$
Subtract Fractions: Subtract the fractions in the exponent. $\newline$ $y^{\frac{10}{15} - \frac{4}{15}} = y^{\frac{6}{15}}$
Simplify Fraction: Simplify the fraction in the exponent. $y^{\frac{6}{15}}$ simplifies to $y^{\frac{2}{5}}$ because $6$ and $15$ have a common factor of $3$ .