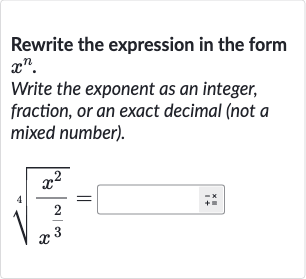
Rewrite the expression in the form x^(n). Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). root(4)((x^(2))/(x^((2)/(3))))=◻^(◻-x)

Question

Rewrite the expression in the form  x^(n). Write the exponent as an integer, fraction, or an exact decimal (not a mixed number).  root(4)((x^(2))/(x^((2)/(3))))=◻^(◻-x)

Bytelearn · Accepted Answer

Identify expression and root type: Identify the expression and the type of root. The expression is root(4)((x^(2))/(x^((2)/(3)))), which means we have a fourth root of a fraction where the numerator is x squared and the denominator is x raised to the power of two-thirds.Rewrite as exponent: Rewrite the fourth root as an exponent. The fourth root of a number can be written as raising that number to the power of 1/4. So, root(4)((x^(2))/(x^((2)/(3)))) can be written as ((x^(2))/(x^((2)/(3))))^(1/4).Simplify expression inside root: Use the properties of exponents to simplify the expression inside the root. When dividing like bases with exponents, we subtract the exponents: x^(a) / x^(b) = x^(a-b). So, (x^(2))/(x^((2)/(3))) simplifies to x^(2 - (2/3)).Calculate exponent: Calculate the exponent. 2 - (2/3) = (6/3) - (2/3) = 4/3. So, (x^(2))/(x^((2)/(3))) simplifies to x^(4/3).Apply fourth root: Apply the fourth root to the simplified expression. Now we have (x^(4/3))^(1/4). When raising a power to a power, we multiply the exponents: (x^(a))^(b) = x^(a*b). So, (x^(4/3))^(1/4) simplifies to x^((4/3)*(1/4)).Calculate new exponent: Calculate the new exponent. (4/3) * (1/4) = 4/12 = 1/3. So, (x^(4/3))^(1/4) simplifies to x^(1/3).Write final answer: Write the final answer. The expression root(4)((x^(2))/(x^((2)/(3)))) rewritten in the form x^(n) is x^(1/3).

Rewrite the expression in the form $x^{n}$ . $\newline$ Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). $\newline$ $\sqrt[4]{\frac{x^{2}}{x^{\frac{2}{3}}}}=\square$

Full solution

More problems from Powers with decimal and fractional bases

Related topics

Rewrite the expression in the form xn x^{n} xn.\newlineWrite the exponent as an integer, fraction, or an exact decimal (not a mixed number).\newlinex2x234=□ \sqrt[4]{\frac{x^{2}}{x^{\frac{2}{3}}}}=\square 4x32​x2​​=□

Rewrite the expression in the form $x^{n}$ . $\newline$ Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). $\newline$ $\sqrt[4]{\frac{x^{2}}{x^{\frac{2}{3}}}}=\square$