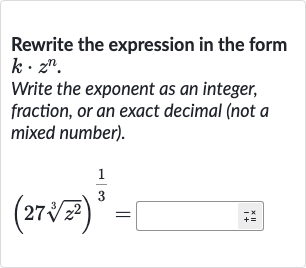
Rewrite the expression in the form k*z^(n). Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). (27root(3)(z^(2)))^((1)/(3))=◻

Question

Rewrite the expression in the form  k*z^(n). Write the exponent as an integer, fraction, or an exact decimal (not a mixed number).  (27root(3)(z^(2)))^((1)/(3))=◻

Bytelearn · Accepted Answer

Identify components: Understand the given expression and identify the components. The given expression is (27root(3)(z^(2)))^((1)/(3)). We need to rewrite this expression in the form k*z^(n), where k is a constant and n is the exponent.Rewrite as exponent: Rewrite the cube root in the expression as an exponent. The cube root of a number can be written as that number raised to the power of 1/3. So, 27root(3)(z^(2)) can be written as (27^(1/3))*(z^(2*(1/3))).Simplify cube root of 27: Simplify the expression for the cube root of 27. Since 27 is 3^3, the cube root of 27 is 3. So, 27^(1/3) simplifies to 3.Simplify exponent for z: Simplify the exponent for z. The exponent for z is 2*(1/3), which simplifies to 2/3. So, z^(2*(1/3)) simplifies to z^(2/3).Apply outer exponent: Apply the outer exponent to the simplified expression. Now we have (3*z^(2/3))^((1)/(3)). When we raise a product to an exponent, we raise each factor to that exponent: 3^((1)/(3)) * (z^(2/3))^((1)/(3)).Simplify 3 raised to 1/3: Simplify the expression for 3 raised to the power of 1/3. Since 3 raised to the power of 1/3 is the cube root of 3, which is just 3, this part of the expression remains 3.Simplify exponent for z: Simplify the exponent for z. When we raise an exponent to another exponent, we multiply the exponents. So, (z^(2/3))^((1)/(3)) simplifies to z^((2/3)*(1/3)), which is z^(2/9).Combine constants and exponent: Combine the constants and the simplified exponent for z. The final expression is 3*z^(2/9).

Rewrite the expression in the form $k \cdot z^{n}$ . $\newline$ Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). $\newline$ $\left(27 \sqrt[3]{z^{2}}\right)^{\frac{1}{3}}=\square$

Full solution

More problems from Powers with decimal and fractional bases

Related topics

Rewrite the expression in the form k⋅zn k \cdot z^{n} k⋅zn.\newlineWrite the exponent as an integer, fraction, or an exact decimal (not a mixed number).\newline(27z23)13=□ \left(27 \sqrt[3]{z^{2}}\right)^{\frac{1}{3}}=\square (273z2​)31​=□

Rewrite the expression in the form $k \cdot z^{n}$ . $\newline$ Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). $\newline$ $\left(27 \sqrt[3]{z^{2}}\right)^{\frac{1}{3}}=\square$