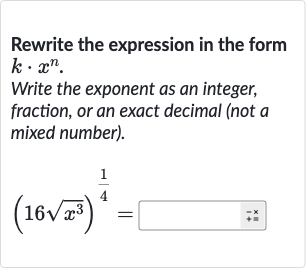
Rewrite the expression in the form k*x^(n). Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). (16sqrt(x^(3)))^((1)/(4))=◻

Question

Rewrite the expression in the form  k*x^(n). Write the exponent as an integer, fraction, or an exact decimal (not a mixed number).  (16sqrt(x^(3)))^((1)/(4))=◻

Bytelearn · Accepted Answer

Simplify inside the parentheses: First, let's simplify the expression inside the parentheses before applying the outer exponent. The expression inside the parentheses is 16sqrt(x^(3)). The square root of x^3 can be written as x^(3/2).Rewrite expression without radicals: Now, we can rewrite the expression as (16 * x^(3/2)). Since there are no square roots or other radicals left inside the parentheses, we can now apply the outer exponent of 1/4 to both the constant and the variable.Apply exponent to constant and variable: Applying the exponent of 1/4 to 16, we get 16^(1/4). The fourth root of 16 is 2, because 2^4 = 16.Calculate exponent of constant: Applying the exponent of 1/4 to x^(3/2), we use the rule of exponents that states (x^a)^b = x^(a*b). So, x^(3/2 * 1/4) = x^(3/8).Combine results for final expression: Combining the results from the previous steps, we get the final expression in the form k*x^(n), which is 2*x^(3/8).

Rewrite the expression in the form $k \cdot x^{n}$ . $\newline$ Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). $\newline$ $\left(16 \sqrt{x^{3}}\right)^{\frac{1}{4}}=\square$

Full solution

More problems from Powers with decimal and fractional bases

Related topics

Rewrite the expression in the form k⋅xn k \cdot x^{n} k⋅xn.\newlineWrite the exponent as an integer, fraction, or an exact decimal (not a mixed number).\newline(16x3)14=□ \left(16 \sqrt{x^{3}}\right)^{\frac{1}{4}}=\square (16x3​)41​=□

Rewrite the expression in the form $k \cdot x^{n}$ . $\newline$ Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). $\newline$ $\left(16 \sqrt{x^{3}}\right)^{\frac{1}{4}}=\square$