AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
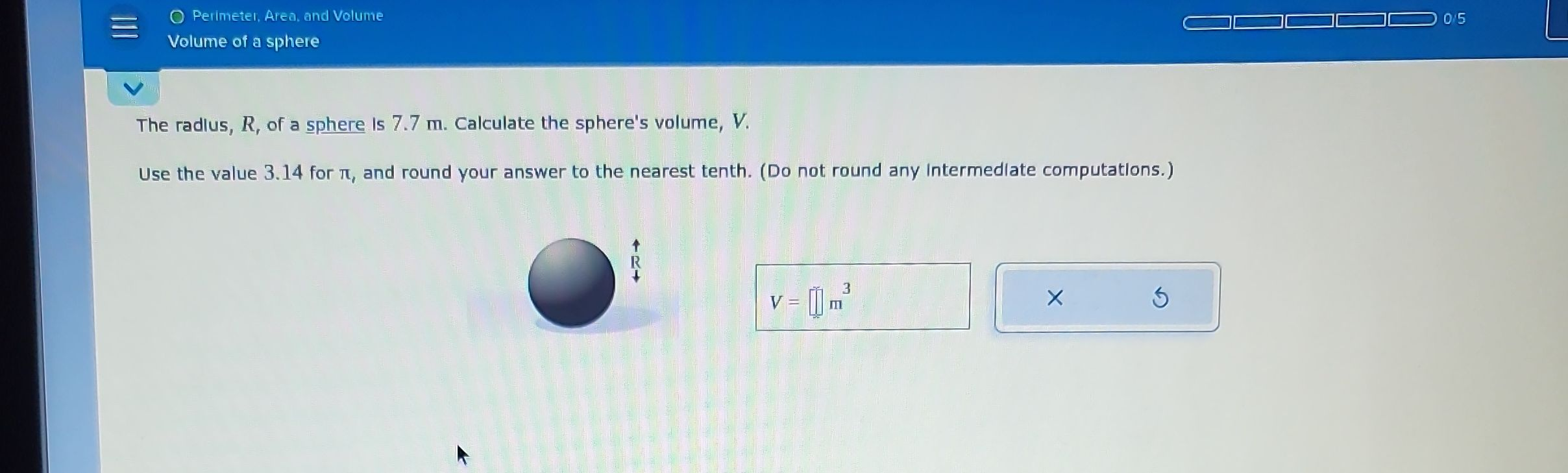
Perimeter, Area, and VolumeVolume of a sphereThe radlus, , of a sphere is . Calculate the sphere's volume, .Use the value . for , and round your answer to the nearest tenth. (Do not round any Intermedlate computations.)
Full solution
Q. Perimeter, Area, and VolumeVolume of a sphereThe radlus, , of a sphere is . Calculate the sphere's volume, .Use the value . for , and round your answer to the nearest tenth. (Do not round any Intermedlate computations.)
- Identify Formula: Identify the formula for the volume of a sphere.The formula for the volume of a sphere is , where is the volume, is pi, and is the radius of the sphere.
- Substitute Values: Substitute the given values into the formula.We are given the radius meters and . So, we substitute these values into the formula to get .
- Calculate Volume: Calculate the volume.First, calculate the radius cubed: .Then, multiply this by : .Finally, multiply by : .
- Round Result: Round the result to the nearest tenth.The volume of the sphere, rounded to the nearest tenth, is cubic meters.
More problems from Evaluate two-variable equations: word problems
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
