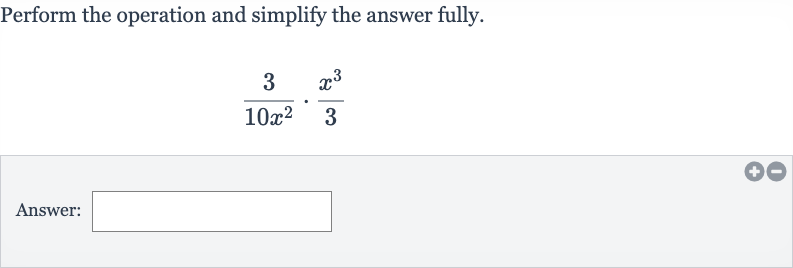Full solution
Q. Perform the operation and simplify the answer fully.Answer:
- Identify Operation: Identify the operation to be performed.We need to multiply two fractions: and .
- Multiply Numerators and Denominators: Multiply the numerators and denominators.When multiplying fractions, we multiply the numerators together and the denominators together.
- Simplify Expression: Simplify the expression by canceling out common factors.The number in the numerator and denominator can be canceled out, and in the denominator can be canceled with two of the 's in in the numerator.
- Perform Subtraction and Simplify: Perform the subtraction in the exponent and simplify the fraction. simplifies to , which is just . The fraction simplifies to .
- Write Final Result: Write the final simplified result.The final simplified result is .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help