Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

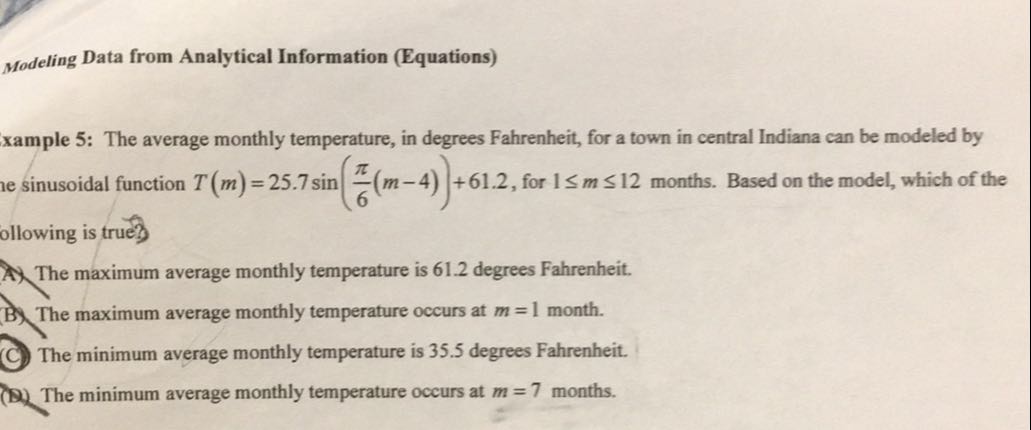
The average monthly temperature, in degrees Fahrenheit, for a town in central Indiana can be modeled by the sinusoidal function T(m)=25.7 sin((pi)/(6)(m-4))+61.2, for 1 <= m <= 12 months. Based on the model, which of the following is true? (A) The maximum average monthly temperature is 61.2 degrees Fahrenheit. (B) The maximum average monthly temperature occurs at m=1 month. (C) The minimum average monthly temperature is 35.5 degrees Fahrenheit. (D) The minimum average monthly temperature occurs at m=7 months.

The average monthly temperature, in degrees Fahrenheit, for a town in central Indiana can be modeled by the sinusoidal function T(m)=25.7sin⁡(π6(m−4))+61.2 T(m)=25.7 \sin \left(\frac{\pi}{6}(m-4)\right)+61.2 , for 1≤m≤12 1 \leq m \leq 12 months. Based on the model, which of the following is true?\newline(A) The maximum average monthly temperature is 6161.22 degrees Fahrenheit.\newline(B) The maximum average monthly temperature occurs at m=1 m=1 month.\newline(C) The minimum average monthly temperature is 3535.55 degrees Fahrenheit.\newline(D) The minimum average monthly temperature occurs at m=7 m=7 months.

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 7right chevron icon
Evaluate two-variable equations: word problemsright chevron icon

Full solution

Q. The average monthly temperature, in degrees Fahrenheit, for a town in central Indiana can be modeled by the sinusoidal function T(m)=25.7sin⁡(π6(m−4))+61.2 T(m)=25.7 \sin \left(\frac{\pi}{6}(m-4)\right)+61.2 , for 1≤m≤12 1 \leq m \leq 12 months. Based on the model, which of the following is true?\newline(A) The maximum average monthly temperature is 6161.22 degrees Fahrenheit.\newline(B) The maximum average monthly temperature occurs at m=1 m=1 month.\newline(C) The minimum average monthly temperature is 3535.55 degrees Fahrenheit.\newline(D) The minimum average monthly temperature occurs at m=7 m=7 months.
  1. Analyze Function: Analyze the given sinusoidal function for temperature.\newlineThe function T(m)=25.7sin⁡(π6(m−4))+61.2T(m) = 25.7 \sin\left(\frac{\pi}{6}(m-4)\right) + 61.2 models the average monthly temperature in degrees Fahrenheit. The general form of a sinusoidal function is T(m)=Asin⁡(B(m−C))+DT(m) = A \sin(B(m - C)) + D, where:\newline- AA is the amplitude (half the distance between the maximum and minimum values),\newline- BB affects the period of the function,\newline- CC is the horizontal shift (which determines where the maximum or minimum occurs),\newline- DD is the vertical shift (which determines the midline of the function).
  2. Determine Amplitude: Determine the amplitude of the function.\newlineThe amplitude is given by the coefficient of the sine function, which is 25.725.7. This means the temperature fluctuates 25.725.7 degrees above and below the midline.
  3. Determine Midline: Determine the midline of the function.\newlineThe midline is given by the constant term added to the sine function, which is 61.261.2. This is the average value around which the temperature fluctuates.
  4. Calculate Maximum Temperature: Calculate the maximum average monthly temperature. The maximum temperature occurs at the midline plus the amplitude. Therefore, the maximum average monthly temperature is 61.2+25.7=86.961.2 + 25.7 = 86.9 degrees Fahrenheit.
  5. Calculate Minimum Temperature: Calculate the minimum average monthly temperature. The minimum temperature occurs at the midline minus the amplitude. Therefore, the minimum average monthly temperature is 61.2−25.7=35.561.2 - 25.7 = 35.5 degrees Fahrenheit.
  6. Determine Maximum Month: Determine when the maximum average monthly temperature occurs.\newlineThe maximum temperature occurs when the sine function is at its peak, which is at sin⁡(π2)\sin(\frac{\pi}{2}). To find the corresponding month (mm), we set the inside of the sine function equal to π2\frac{\pi}{2} and solve for mm:\newline(π6)(m−4)=π2\left(\frac{\pi}{6}\right)(m-4) = \frac{\pi}{2}\newlinem−4=3m - 4 = 3\newlinem=7m = 7\newlineTherefore, the maximum average monthly temperature occurs at m=7m=7 months.
  7. Determine Minimum Month: Determine when the minimum average monthly temperature occurs.\newlineThe minimum temperature occurs when the sine function is at its lowest, which is at sin⁡(3π2)\sin(\frac{3\pi}{2}). To find the corresponding month (mm), we set the inside of the sine function equal to 3π2\frac{3\pi}{2} and solve for mm:\newlineπ6(m−4)=3π2\frac{\pi}{6}(m-4) = \frac{3\pi}{2}\newlinem−4=9m - 4 = 9\newlinem=13m = 13\newlineHowever, since the model is only valid for 1≤m≤121 \leq m \leq 12, we need to find the equivalent value within this range. Since the sine function has a period of 1212 months, we subtract 1212 from 1313 to find the equivalent month within the valid range:\newlinem=13−12m = 13 - 12\newlinem=1m = 1\newlineTherefore, the minimum average monthly temperature occurs at mm00 month.

More problems from Evaluate two-variable equations: word problems

Question
This equation shows how the distance traveled by a car is related to the time spent driving. d=50t+10 d = 50t + 10 . The variable t t represents the time in hours, and the variable d d represents the distance in miles. How far does the car travel in 2 2 hours?
Get tutor helpright-arrow

Posted 10 months ago

Question
The equation shows how the cost of buying pencils is related to the number of pencils purchased. \newline c=0.5p+2 c= 0.5p+2 \newlineThe variable p p represents the number of pencils, and the variable c c represents the total cost. How much does it cost to buy 10 10 pencils?
Get tutor helpright-arrow

Posted 9 months ago

Question
This equation shows how the total score in a game is related to the number of points scored per level. s=100l+250 s = 100l + 250 . The variable l l represents the levels completed, and the variable s s represents the total score. What is the total score after completing 3 3 levels?
Get tutor helpright-arrow

Posted 7 months ago

Question
The equation shows how the amount of paint needed is related to the area to be painted. p=2a+5 p = 2a + 5 . The variable a a represents the area in square meters, and the variable p p represents the amount of paint in liters. How much paint is needed for an area of 8 8 square meters?
Get tutor helpright-arrow

Posted 7 months ago

Question
This equation shows how the total bill for a meal is related to the number of people sharing the meal. \newline b=15n+35 b = 15n + 35 \newlineThe variable n n represents the number of people, and the variable b b represents the total bill. What is the total bill for a meal shared by 4 4 people?
Get tutor helpright-arrow

Posted 9 months ago

Question
Poppy the dog weighs p p pounds, and Yumi the cat weighs y y pounds. If Poppy weighs 3636 more pounds than Yumi does, which of the following equations correctly describes the relationship between their weights?\newlineChoose 11 answer:\newline(A) p+y=36 p+y=36 \newline(B) p+36=y p+36=y \newline(C) p−y=36 p-y=36 \newline(D) y−p=36 y-p=36
Get tutor helpright-arrow

Posted 7 months ago

Question
Calvin and Melvin are two of Santa's elves. The number of toys they build is given by 11c+8m 11 c+8 m , where c c is the number of candy canes Calvin eats for breakfast and m m is the number of candy canes Melvin eats for breakfast. How many toys do they build when Calvin eats 55 candy canes and Melvin eats 33 candy canes for breakfast?
Get tutor helpright-arrow

Posted 7 months ago

Question
2w+4 2 w+4 \newlineCarly is cutting a piece of paper stock based on the width of a photo. The expression above describes the length of the paper stock, in inches, for a photo that is w w inches wide. If the photo is 88.55 inches wide, what is the length of the paper stock in inches?
Get tutor helpright-arrow

Posted 7 months ago

Question
Calvin and Melvin are two of Santa's elves. The number of toys they build is given by 11c+8m 11 c+8 m where c c is the number of candy canes Calvin eats for breakfast and m m is the number of candy canes Melvin eats for breakfast.\newlineHow many toys do they build when Calvin eats 55 candy canes and Melvin eats 33 candy canes for breakfast?\newlinetoys
Get tutor helpright-arrow

Posted 8 months ago

Question
The approval rating of a certain politician is changing at a rate of r(t) r(t) percent of the population per month (where t t is the time in months).\newlineWhat does ∫03r(t)dt \int_{0}^{3} r(t) d t represent?\newlineChoose 11 answer:\newline(A) The rate of change of the approval rating of the politician when t=3 t=3 months\newline(B) The average rate of change of the approval rating during the first three months\newline(C) The change in the percent of the population who approves of the politician during the first three months\newline(D) The percent of the population who approves of the politician when t=3 t=3 months
Get tutor helpright-arrow

Posted 7 months ago

View all (180)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant