AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
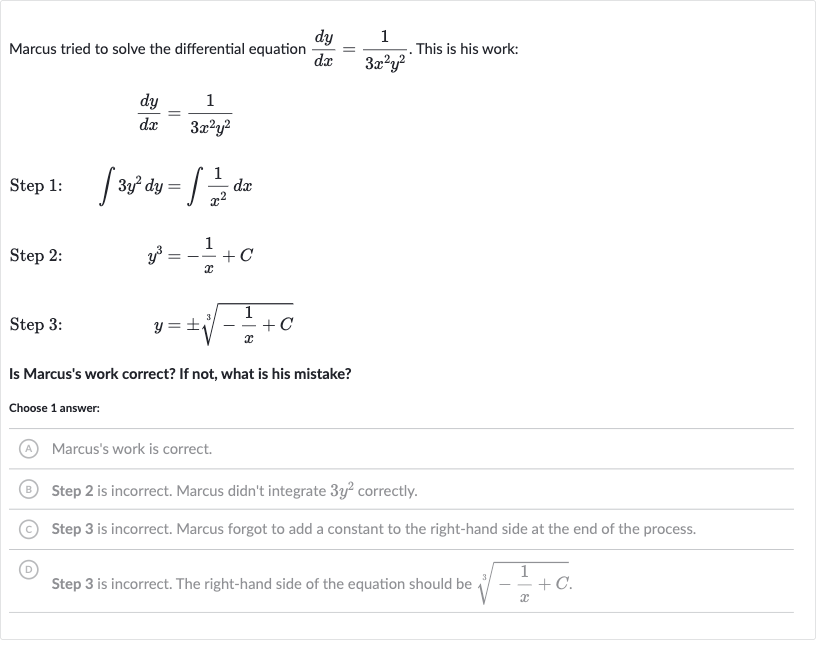
Marcus tried to solve the differential equation . This is his work:Step : Step : Step : Is Marcus's work correct? If not, what is his mistake?Choose answer:(A) Marcus's work is correct.(B) Step is incorrect. Marcus didn't integrate correctly.(C) Step is incorrect. Marcus forgot to add a constant to the right-hand side at the end of the process.(D) Step is incorrect. The right-hand side of the equation should be .
Full solution
Q. Marcus tried to solve the differential equation . This is his work:Step : Step : Step : Is Marcus's work correct? If not, what is his mistake?Choose answer:(A) Marcus's work is correct.(B) Step is incorrect. Marcus didn't integrate correctly.(C) Step is incorrect. Marcus forgot to add a constant to the right-hand side at the end of the process.(D) Step is incorrect. The right-hand side of the equation should be .
- Separate and Integrate Variables: Marcus starts by separating variables and integrating both sides of the differential equation.Multiplying both sides by dx and gives:Now, integrate both sides:
- Multiply and Integrate: Marcus integrates both sides of the equation.The integral of with respect to is , not , because the integral of with respect to is when .So, , where is the constant of integration.On the right side, the integral of with respect to is , where is the constant of integration.Therefore, the correct integration should be:
