AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
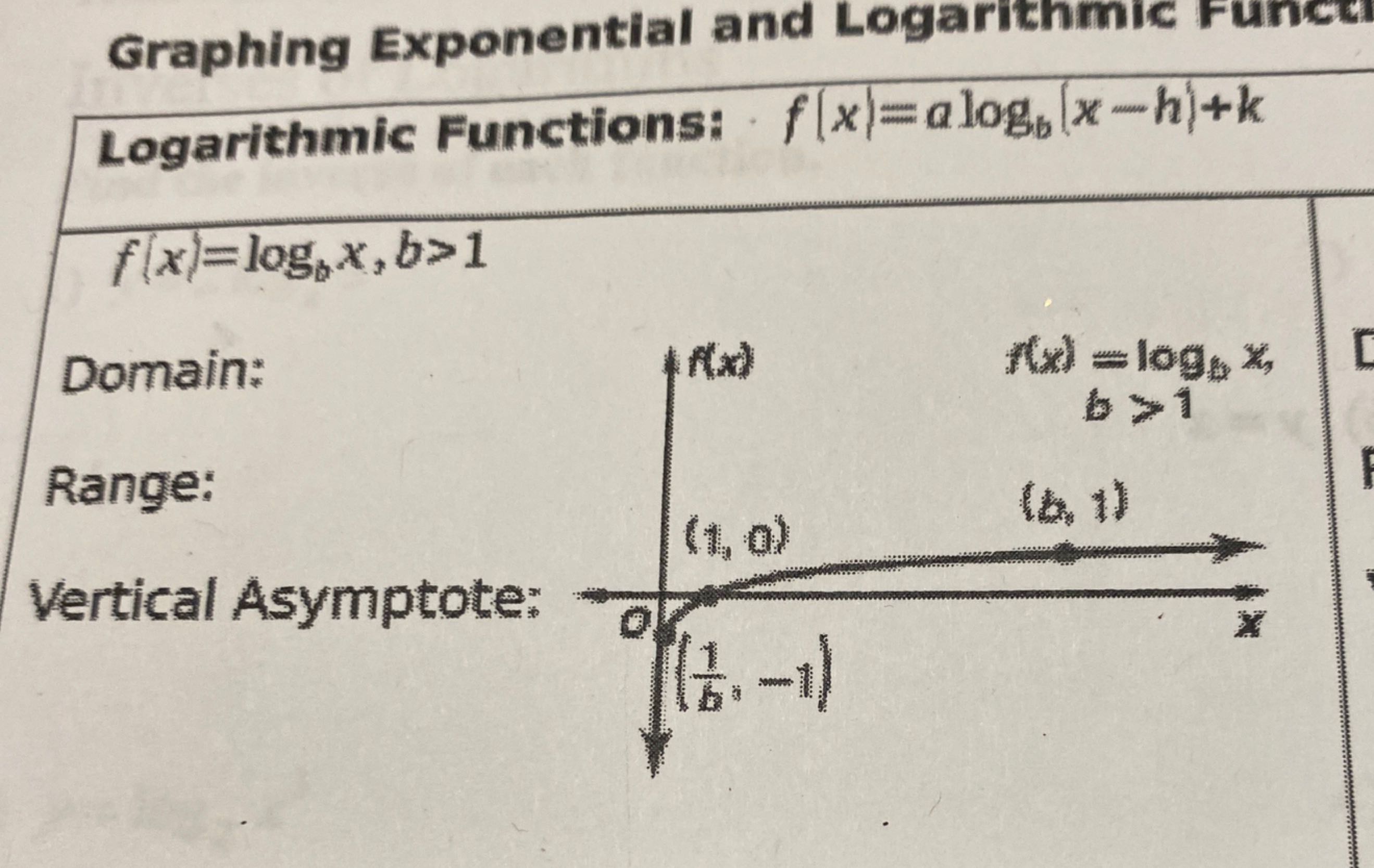
Logarithmic Functions: , b > 1Domain:Range:Vertical Asymptote:
Full solution
Q. Logarithmic Functions: , Domain:Range:Vertical Asymptote:
- Identify Base Impact: Identify the base of the logarithm and its impact on the function.Since b > 1, the base is a valid positive number not equal to , which is necessary for the logarithmic function to be defined.
- Find Domain: Find the domain of . The logarithmic function is defined only for x > 0. Therefore, the domain of is all positive real numbers.
- Determine Range: Determine the range of . The output of a logarithmic function can be any real number, which means the range of is all real numbers.
- Identify Vertical Asymptote: Identify the vertical asymptote of . The vertical asymptote occurs where the function is undefined and near which the function heads towards negative or positive infinity. For , this is at .
