Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

log_(81)(1)/(27)=

$\log _{81} \frac{1}{27}=$

View step-by-step help

View step-by-step help

Evaluate logarithms using properties

Full solution

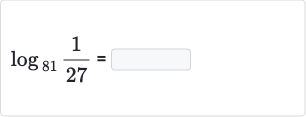
Q.

\log _{81} \frac{1}{27}=

Recognize base and argument: Recognize the base of the logarithm and the argument. The base of the logarithm is $81$ , and the argument is $\frac{1}{27}$ . We need to express $\frac{1}{27}$ as a power of $81$ .
Express as power of $81$ : Express $\frac{1}{27}$ as a power of $81$ . $\newline$ Since $81$ is $3^4$ and $27$ is $3^3$ , we can write $\frac{1}{27}$ as $(3^4)^{-\frac{1}{3}}$ because $(3^4)^{\frac{1}{3}} = 3^{\frac{4}{3}} = 27$ .
Rewrite using new expression: Rewrite the logarithm using the new expression for $\frac{1}{27}$ . $\newline$ $\log_{81}\left((3^4)^{-\frac{1}{3}}\right)$
Apply change of base formula: Apply the change of base formula for logarithms. $\newline$ The change of base formula is $\log_b(a) = \frac{\log_c(a)}{\log_c(b)}$ . In this case, we can use base $3$ for both the numerator and the denominator. $\newline$ $\log_{81}((3^4)^{-\frac{1}{3}}) = \frac{\log_3((3^4)^{-\frac{1}{3}})}{\log_3(81)}$
Simplify logarithms: Simplify the logarithms. $\newline$ Since $81$ is $3^4$ , $\log_3(81) = 4$ . The numerator is $\log_3((3^4)^{-1/3})$ , which simplifies to $-\frac{1}{3} \times \log_3(3^4)$ because of the power property of logarithms.
Simplify numerator further: Simplify the numerator further. $\newline$ $-\frac{1}{3} \times \log_3(3^4)$ simplifies to $-\frac{1}{3} \times 4$ because $\log_3(3^4) = 4$ .
Calculate expression value: Calculate the value of the expression. $\newline$ $-\frac{1}{3} \times 4 / 4$ simplifies to $-\frac{1}{3}$ .
Conclude final answer: Conclude the final answer. $\newline$ The value of $\log_{81}(\frac{1}{27})$ is $-\frac{1}{3}$ .

More problems from Evaluate logarithms using properties

Question

\newline

\frac{5}{6} \div \frac{9}{4}=

Posted 3 months ago

Question

\newline

\frac{8}{3} \div \frac{5}{3}=

Posted 3 months ago

Question

\newline

\frac{5}{4} \div \frac{3}{5}=

Posted 3 months ago

Question

\newline

\frac{3}{4} \div \frac{3}{4}=

Posted 3 months ago

Question

Write the expression in exponential form.

\newline

6 \times 6 \times 6 \times 6 \times 6=

Posted 3 months ago

Question

Write the expression in exponential form.

\newline

9 \times 9 \times 9 \times 9 \times 9 \times 9 \times 9=

Posted 3 months ago

Question

The value of Vishal's car is depreciating exponentially.

\newline

The relationship between

V

, the value of his car, in dollars, and

t

, the elapsed time, in years, since he purchased the car is modeled by the following equation.

\newline

V=22,500 \cdot 10^{-\frac{t}{12}}

\newline

How many years after purchase will Vishal's car be worth

\$ 10,000

\newline

Give an exact answer expressed as a base

-10

\newline

Posted 3 months ago

Question

A scientist measures the initial amount of Carbon

-14

in a substance to be

25

\newline

The relationship between

A

, the amount of Carbon

-14

remaining in that substance, in grams, and

t

, the elapsed time, in years, since the initial measurement is modeled by the following equation.

\newline

A=25 e^{-0.00012 t}

\newline

In how many years will the substance contain exactly

20

(\mathrm{g})

-14

\newline

Give an exact answer expressed as a natural logarithm.

\newline

Posted 3 months ago

Question

Which of the following is equivalent to

\log _{7}(a) \cdot \log _{b}(7)

\newline

1

\newline

\log (7)

\newline

\log (7 a)

\newline

\log _{a}(b)

\newline

\log _{b}(a)

Posted 3 months ago

Question

Which of the following is equivalent to

\log (a) \cdot \log _{a}(5)

\newline

1

\newline

\log (a)

\newline

\log (5)

\newline

\log (5 a)

\newline

\log _{a}(5 a)

Posted 3 months ago