Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

log_(10)1,000=

log⁡101,000= \log _{10} 1,000=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Convert between exponential and logarithmic form: all basesright chevron icon

Full solution

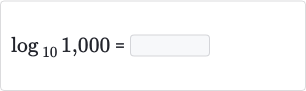
Q. log⁡101,000= \log _{10} 1,000=
  1. Identify base, number, exponent: Identify the base bb, the number xx, and the exponent yy that the logarithm is equal to.\newlineIn the logarithmic equation log⁡101000\log_{10}1000, the base bb is 1010 and the number xx is 10001000. We need to find the exponent yy such that 10y=100010^y = 1000.
  2. Recognize power of 1010: Recognize that 1,0001,000 is a power of 1010. Specifically, 1,0001,000 is 10310^3 because 10×10×10=1,00010 \times 10 \times 10 = 1,000.
  3. Write exponential form: Write the exponential form of the logarithmic equation. Since we know that 103=1,00010^3 = 1,000, the logarithmic equation log⁡101,000\log_{10}1,000 is equal to 33.
  4. Confirm equivalence: Confirm that the exponential form matches the original logarithmic equation. The logarithmic equation log⁡101000=3\log_{10}1000 = 3 is equivalent to the exponential equation 103=1,00010^3 = 1,000.

More problems from Convert between exponential and logarithmic form: all bases

Question
Evaluate.\newline56÷94= \frac{5}{6} \div \frac{9}{4}=
Get tutor helpright-arrow

Posted 4 months ago

Question
Evaluate.\newline83÷53= \frac{8}{3} \div \frac{5}{3}=
Get tutor helpright-arrow

Posted 4 months ago

Question
Evaluate.\newline54÷35= \frac{5}{4} \div \frac{3}{5}=
Get tutor helpright-arrow

Posted 4 months ago

Question
Evaluate.\newline34÷34= \frac{3}{4} \div \frac{3}{4}=
Get tutor helpright-arrow

Posted 4 months ago

Question
Write the expression in exponential form.\newline6×6×6×6×6= 6 \times 6 \times 6 \times 6 \times 6=
Get tutor helpright-arrow

Posted 4 months ago

Question
Write the expression in exponential form.\newline9×9×9×9×9×9×9= 9 \times 9 \times 9 \times 9 \times 9 \times 9 \times 9=
Get tutor helpright-arrow

Posted 4 months ago

Question
The value of Vishal's car is depreciating exponentially.\newlineThe relationship between V V , the value of his car, in dollars, and t t , the elapsed time, in years, since he purchased the car is modeled by the following equation.\newlineV=22,500⋅10−t12 V=22,500 \cdot 10^{-\frac{t}{12}} \newlineHow many years after purchase will Vishal's car be worth $10,000 \$ 10,000 ?\newlineGive an exact answer expressed as a base−10-10 logarithm.\newlineyears
Get tutor helpright-arrow

Posted 4 months ago

Question
A scientist measures the initial amount of Carbon−14-14 in a substance to be 2525 grams.\newlineThe relationship between A A , the amount of Carbon−14-14 remaining in that substance, in grams, and t t , the elapsed time, in years, since the initial measurement is modeled by the following equation.\newlineA=25e−0.00012t A=25 e^{-0.00012 t} \newlineIn how many years will the substance contain exactly 2020 grams (g) (\mathrm{g}) of Carbon−14-14?\newlineGive an exact answer expressed as a natural logarithm.\newlineyears
Get tutor helpright-arrow

Posted 4 months ago

Question
Which of the following is equivalent to log⁡7(a)⋅log⁡b(7) \log _{7}(a) \cdot \log _{b}(7) ?\newlineChoose 11 answer:\newline(A) log⁡(7) \log (7) \newline(B) log⁡(7a) \log (7 a) \newline(C) log⁡a(b) \log _{a}(b) \newline(D) log⁡b(a) \log _{b}(a)
Get tutor helpright-arrow

Posted 4 months ago

Question
Which of the following is equivalent to log⁡(a)⋅log⁡a(5) \log (a) \cdot \log _{a}(5) ?\newlineChoose 11 answer:\newline(A) log⁡(a) \log (a) \newline(B) log⁡(5) \log (5) \newline(C) log⁡(5a) \log (5 a) \newline(D) log⁡a(5a) \log _{a}(5 a)
Get tutor helpright-arrow

Posted 4 months ago

View all (14)