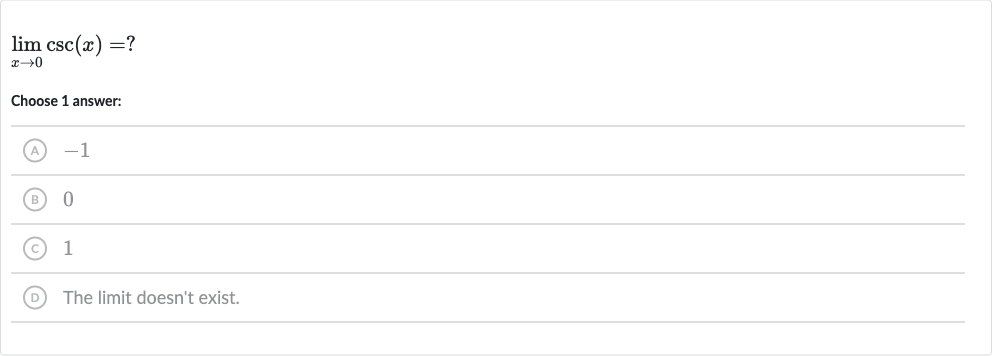
Full solution
Q. Choose answer:(A) (B) (C) (D) The limit doesn't exist.
- Definition of : To solve the limit of as approaches , we need to understand the definition of , which is . We are looking for the limit of as approaches .
- Behavior of sin(x) as x approaches : We know that . As approaches , approaches as well. Therefore, we are essentially looking at the behavior of as approaches .
- Undefined expression : The expression is undefined, which means that as gets closer and closer to , (or ) grows without bound. This suggests that the limit does not exist because the function does not approach a finite value.
- Limit of as approaches : Since the limit of as approaches does not approach a finite value and instead grows without bound, the correct answer is (D) The limit doesn't exist.