Full solution
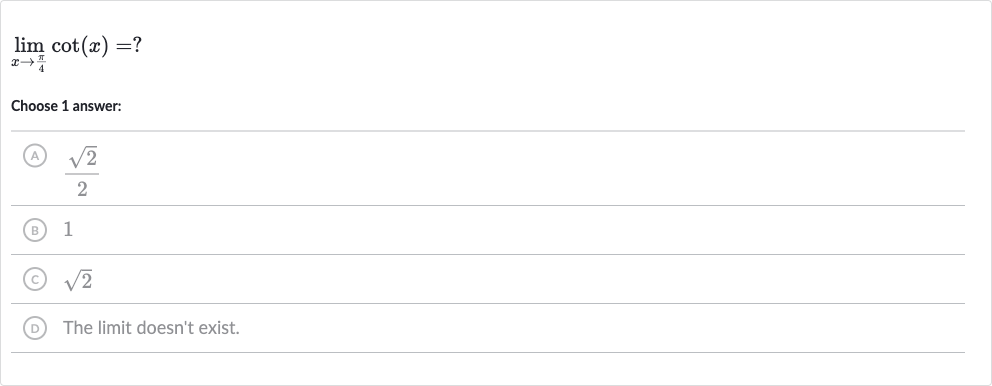
Q. Choose answer:(A) (B) (C) (D) The limit doesn't exist.
- Recalling the definition of cotangent: First, let's recall the definition of cotangent in terms of sine and cosine:We will use this definition to find the limit as approaches .
- Evaluating cosine and sine: Now, let's evaluate the cosine and sine of :These are well-known values from the unit circle.
- Substituting values into cotangent expression: Next, we substitute these values into the cotangent expression:
- Simplifying the expression: We simplify the expression by dividing the numerators and the denominators:
- Concluding the limit: Since the limit of as approaches is simply the value of , we conclude that: