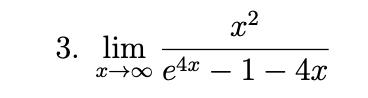AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
Evaluate the limit
Full solution
Q. Evaluate the limit
- Identify Form of Limit: Identify the form of the limit.We need to determine the form of the limit as approaches infinity for the function . By substituting with infinity, we can see that the numerator grows as a polynomial of degree , while the denominator grows exponentially. Since exponential functions grow faster than polynomial functions, we expect the limit to be . However, we need to confirm this by further analysis.
- Apply L'Hôpital's Rule: Apply L'Hôpital's Rule.Since the limit as approaches infinity seems to be of the form [], we can apply L'Hôpital's Rule. This rule states that if the limit of as approaches a value is [] or [], then the limit is the same as the limit of the derivatives of the numerator and the denominator. We will differentiate the numerator and the denominator separately.
- Differentiate Numerator: Differentiate the numerator.The derivative of with respect to is .
- Differentiate Denominator: Differentiate the denominator.The derivative of with respect to is .
- Apply L'Hôpital's Rule with Derivatives: Apply L'Hôpital's Rule by substituting the derivatives.Now we have a new limit to evaluate: . We can simplify this by dividing both the numerator and the denominator by , which gives us .
- Evaluate New Limit: Evaluate the new limit.As approaches infinity, the numerator grows linearly, while the denominator still grows exponentially. Therefore, the limit of as approaches infinity is .
