Full solution
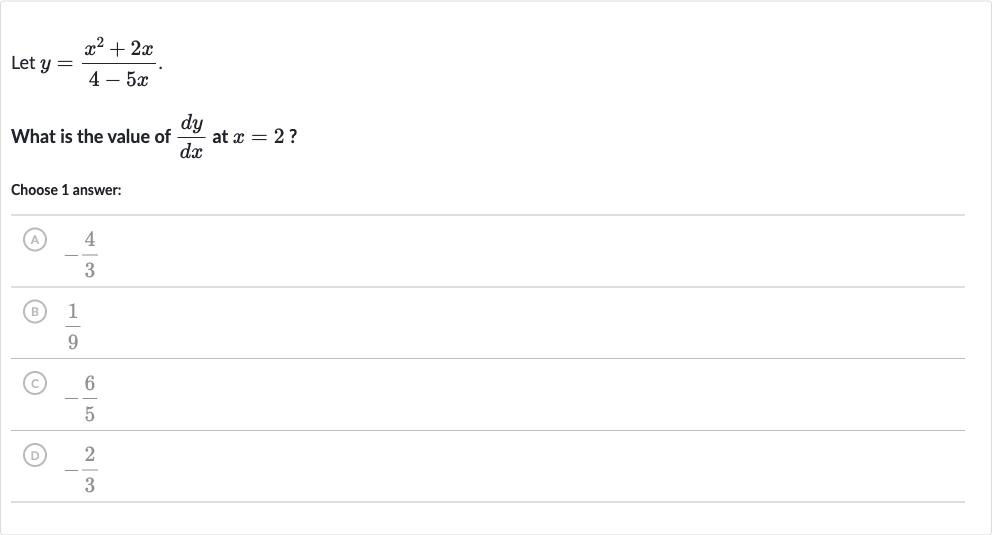
Q. Let .What is the value of at Choose answer:(A) (B) (c) (D)
- Differentiate Function: Differentiate the function with respect to . We have . To find , we need to use the quotient rule for differentiation, which is , where is the numerator and is the denominator of the function.
- Identify and : Identify and and their derivatives.Let and . Then, the derivatives are and .
- Apply Quotient Rule: Apply the quotient rule.Using the quotient rule, .
- Simplify Expression: Simplify the expression....
- Plug in : Plug in into the simplified expression of .
at is .
at is .
at is .
at is .
at is .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help