AI tutor
Full solution
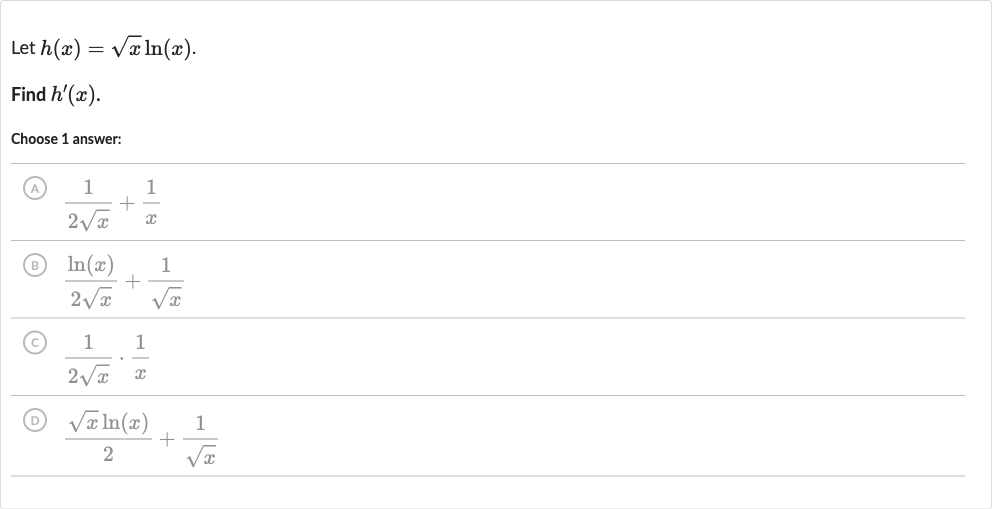
Q. Let .Find .Choose answer:(A) (B) (C) (D)
- Differentiate : Differentiate with respect to to get .Differentiate with respect to to get .
- Differentiate : Now apply the product rule: . This gives us .
- Apply product rule: Simplify the expression: . Oops, there's a mistake here. The second term should be without the .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help