AI tutor
Full solution
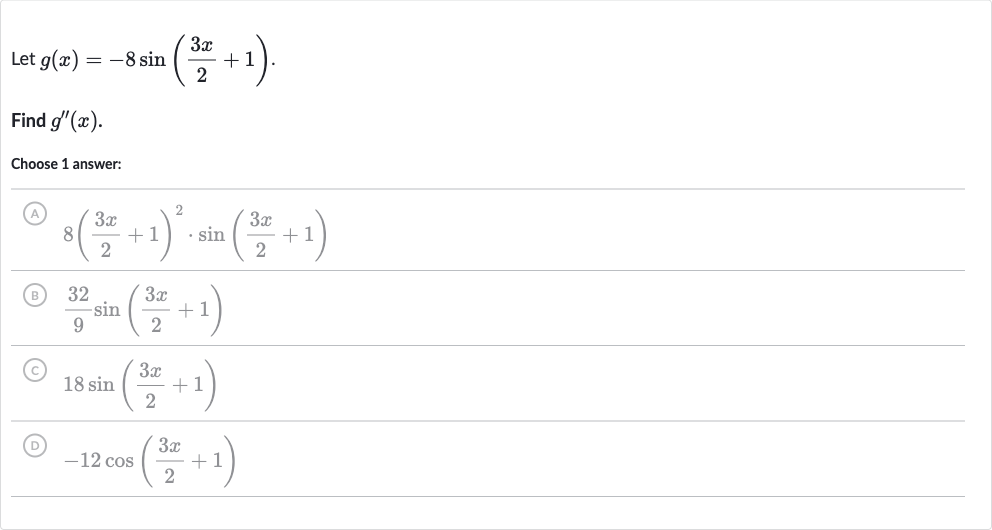
Q. Let .Find .Choose answer:(A) (B) (C) (D)
- Find First Derivative: Differentiate with respect to to find the first derivative .
Using the chain rule, the derivative of with respect to is , where .
The derivative of with respect to is . - Calculate : Differentiate to find the second derivative .
Using the chain rule again, the derivative of with respect to is , where .
The derivative of with respect to is .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help