AI tutor
Full solution
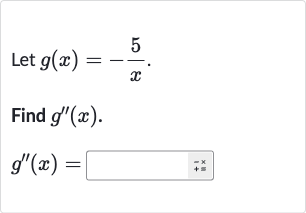
Q. Let .Find .
- Find Derivative of : Find the first derivative of . To find the first derivative of , we use the power rule for derivatives. The function can be rewritten as . Differentiating with respect to gives us .
- Simplify First Derivative: Simplify the first derivative.The first derivative simplifies to .
- Find Second Derivative: Find the second derivative of .Now we need to differentiate to find the second derivative. This can be rewritten as .Differentiating with respect to gives us .
- Simplify Second Derivative: Simplify the second derivative.The second derivative simplifies to .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help